What does it mean for a space to be curved? If it is the space itself which is curved, rather than just the objects within that space, then it is the definition of straightness itself which is curved in that space. In other words if the space were filled with a set of grid-lines marking straight lines with uniform spacing, those lines themselves would be curved rather than straight, as they are in Euclidean space. However the curvature would not be apparent to creatures who live in that curved space, because the curves that are followed by those grid lines are the very definition of straightess in that space. In other words a curved object in that curved space would be defined as perfectly straight, as long as the curvature of the object exactly matched the curvature of the space it was in. If you are having difficulty picturing this paradoxical concept, and suspect that it embodies a contradiction in terms, just look at phenomenal perspective which has exactly that paradoxical property. For phenomenal perspective embodies exactly that same contradiction in terms, with parallel lines meeting at two points while passing to either side of the percipient, and while being perceived to be straight and parallel and equidistant throughout their length.
This absurd contradiction is clearly not a property of the physical world, which is measurably Euclidean at least at the familiar scale of our everyday environment. Therefore that curvature must be a property of perceived space, thereby confirming that perceived space is not the same as the external space of which it is an imperfect replica.
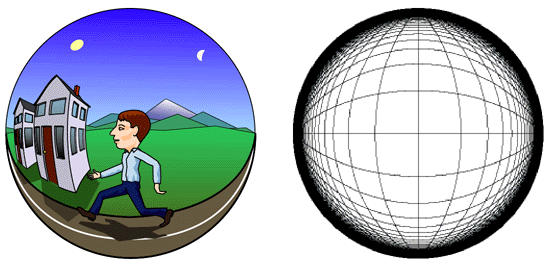
In fact, the observed warping of perceived space is exactly the property that allows the finite representational space to encode an infinite external space. This property is achieved by using a variable representational scale, i.e. the ratio of the physical distance in the perceptual representation relative to the distance in external space that it represents. This scale is observed to vary as a function of distance from the center of our perceived world, such that objects close to the body are encoded at a larger representational scale than objects in the distance, and beyond a certain limiting distance the representational scale, at least in the depth dimension, falls to zero, i.e. objects beyond a certain distance lose all perceptual depth. This is seen for example where the sun and moon and distant mountains appear as if cut out of paper and pasted against the dome of the sky.
This view of perspective offers an explanation for another otherwise paradoxical but familiar property of perceived space whereby more distant objects are perceived to be both smaller, and yet at the same time to be perceived as undiminished in size. This corresponds to the difference in subject's reports depending on whether they are given objective v.s. projective instruction (Coren et al., 1994. p. 500) in how to report their observations, showing that both types of information are available perceptually. This duality in size perception is often described as a cognitive compensation for the foreshortening of perspective, as if the perceptual representation of more distant objects is indeed smaller, but is somehow labeled with the correct size as some kind of symbolic tag representing objective size attached to each object in perception. However this kind of explanation is misleading, for the objective measure of size is not a discrete quantity attached to individual objects, but is more of a continuum, or gradient of difference between objective and projective size, that varies monotonically as a function of distance from the percipient. In other words, this phenomenon is best described as a warping of the space itself within which the objects are represented, so that objects that are warped coherently along with the space in which they are embedded appear undistorted perceptually.
Psychophysical evidence for the curvature of perceived space can be found in the Hallway Experiment.