Plato's Cave: Non-Collinear Illusory Grouping
Non-Collinear Illusory Grouping
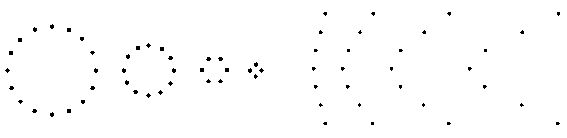
Kanizsa [] shows that when curves defined by lines of dots are given
excessive curvature, the percept of a smooth colinear grouping breaks
into a percept of straight line segments joined by sharp vertices
located at the dots, as shown below, where circles of dots of
increasing curvature begin to appear as polygons. A similar effect is
observed when a line of dots exceeds a critical curvature.
These phenomena indicate that illusory boundary formation can occur in
two modes, either completing smooth "colinear" curves, or through
sharp vertices. The gradual transition between these two modes however
suggests that a similar mechanism underlies both types of boundary
completion.
Besides producing a sharp kink in a linear boundary, illusory boundary
completion can also occur through vertices defined by multiple
orientations in a large range of combinations, as shown below.

Patterns A, B, C, and D shows
arrangements of dots that produce illusory boundaries through vertices
defined by one, two, three, and four dots respectively. A distance
dependent relationship is seen in the grouping patterns of these dots,
where the pattern defined by the nearest neighboring dots determines
the percept of the vertex, and masks the groupings defined by more
remote dots. For example, in B the horizontal
grouping is masked by the stronger vertical grouping because the
vertical distances are smaller than the horizontal ones. If alternate
rows of B were removed, the horizontal grouping would
emerge, showing that the horizontally adjacent dots are sufficiently
close to generate a horizontal grouping, but that that grouping is
masked by the nearer vertical grouping. Similarly, in
C there are three nearest neighbors for each dot,
which defines a three-way vertex passing through every dot in this
pattern. If rows 2, 3, 6, 7, and 10 were removed from this pattern, a
strong vertical grouping would immediately appear. Similarly, notice
how pattern D promotes a four-way grouping at each
dot, suppressing an alternative diagonal grouping between the dots.
These very specific properties observed in illusory vertex phenomena
offer a means to test any proposed model of illusory vertex
completion.
Return to argument
Return to Steve Lehar


