Plato's Cave: Orientational Harmonic Simulations

Computer Simulations of Orientational Harmonics

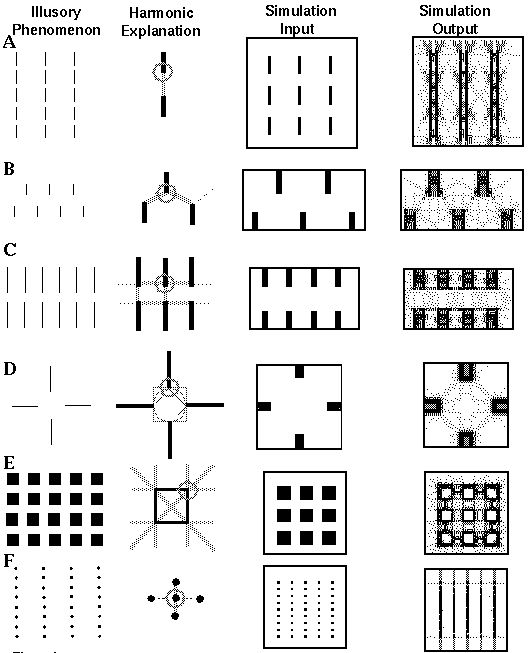
The Orientational Harmonic theory makes specific predictions about interactions between local oriented edges, and about the global emergent patterns resulting from specific input stimuli. Consider for example the pattern of vertical lines depicted in the first column of Figure 6 (A). In the following discussion we will consider only the first four harmonics of oscillation, which comprise the most significant response of the system since higher harmonics in a resonating system tend to be suppressed due to higher impedance at higher frequencies. Consider a point at the bottom end of one of the lines in the figure, circled in the magnified view shown in the second column of Figure 6 (A). The pattern of activation in the cooperative ring at that location would consist of a strong input at the twelve o'clock orientation. The first harmonic response to this input pattern would produce a peak at twelve o'clock, and a negative peak, or suppression of activation at six o'clock. The second harmonic response to this same input pattern would attempt to complete the pattern as a colinear edge, with positive peaks at twelve and six o'clock, and negative peaks at three and nine o'clock. The third harmonic response to this same input would consist of peaks at twelve, four, and eight o'clock with negative peaks in between, and the fourth harmonic response would exhibit positive peaks at six, twelve, three, and nine o'clock. Figure 5 (C) illustrates the pattern of these four harmonics. All four of these harmonic responses would be partially stimulated by the input at twelve o'clock, although the first harmonic would find the closest match, and thus would produce the strongest response. The different harmonics are in a dynamic balance with one another by constructive and destructive interference between wave forms in the orientational representation. For example the positive peak of the second harmonic at six o'clock is balanced by the negative peak of the first harmonic at that orientation. Also the negative peaks of the second harmonic at three and nine o'clock balance the positive peaks of the fourth harmonic at those same orientations. An increase in any one harmonic will therefore affect the dynamic balance between all of the harmonics. For example, the presence of a nearby vertical edge at the six o'clock orientation will produce a weak activation at that orientation due to the distant influence through the cooperative receptive fields which, in conjunction with the strong peak at twelve o'clock would promote the second harmonic or colinear grouping percept in the vertical direction. This in turn would suppress the first and the fourth harmonics, as well as the third harmonic, resulting in a predominantly colinear percept in the form of a vertical grouping percept. The third and fourth columns of Figure 6 (A) depict the input and output respectively of a computer simulation of this phenomenon. Unlike the somewhat artificial simulations of the Directed Diffusion model, these simulations were performed with a spatial convolution of the input image using a set of orientation specific edge filters, calculated as described in the appendix. An orientational harmonic ring was calculated at every pixel location, and the fourth column of Figure 6 represents the equilibrium activation of the system for all orientations combined, a dark shade of grey representing regions of high activation at one or more orientations. The darkest shades are seen in regions corresponding to a direct input, for example along the edges of the vertical lines in the third column of Figure 6 (A). Note that a single line in the computer simulation input corresponds to a double line in the simulation output, representing the dark/light and light/dark edges on either side of that line. The lighter shaded lines in the simulation output which do not correspond to edges in the input image represent illusory boundaries or grouping lines stimulated by that input. In the last column of Figure 6 (A) for instance a strong vertical shading is observed, corresponding to the strong vertical grouping percept observed in the figure. Notice also the weaker diagonal and horizontal lines in the simulation output, repre senting the much weaker third and fourth harmonic responses respectively. By symmetry, the harmonic explanation described for the bottom end of each vertical line applies equally to the top ends of the lines where the same harmonic patterns occur upside down. The grouping of vertical lines depicted in Figure 6 (B) promotes a weak diagonal grouping percept, or an illusory zig-zag edge between the line endings. This corresponds to the third harmonic response since the vertex located at the bottom of each line receives distant activation from the four and eight o'clock orientations as shown in the magnified view in the second col umn of Figure 6 (B), which is consistent with the third harmonic pattern. This in turn suppresses the second and fourth harmonic responses. The computer simulation output for this figure exhib its this diagonal grouping, although the effect is rather weak. This is consistent with the fact that the diagonal grouping percept itself is rather weak. Figure 6 (C) illustrates a horizontal grouping of vertical lines due to the promotion of the fourth harmonic, because the bottom of each line ending receives input both from the six o'clock orientation and from three and nine o'clock, due to fourth harmonic responses at horizontally neighboring line endings. This simulation illustrates the Gestalt concept of global emergent properties from local interactions, because there are initially no inputs from three and nine o'clock, these inputs are only a secondary effect of an emergent fourth harmonic response at all the line endings simultaneously. Figure 6 (C) shows a computer simulation of this phenomenon exhibiting a strong horizontal grouping percept orthogonal to the line endings. A similar orthogonal grouping emerges from a single set of parallel line endings due to fourth harmonic group ing, as is seen in the Ehrenstein illusion shown in Figure 4 (E). The effect is also seen in attenuated form at the top and bottom of Figure 6 (A), and also Figure 6 (F), although it is much suppressed by the second harmonic at the center of those figures. A special case of the Ehrenstein figure is shown in Figure 6 (D) which is reported by subjects [3] to produce either an illusory circle, square, or diamond figure. This illusion therefore appears to rest on a saddle point in perceptual space, which can be perturbed in one of three stable directions. The appearance of any one of the illusory figures however precludes the appearance of the other two. The Orientational Harmonic model explains all of these phenomena by way of a competition, or destructive interference between the second, third, and fourth harmonic waveforms. The second harmonic promotes a colinear completion of the illusory contour orthogonal to the line ending, corresponding to the circular illusory percept; the third harmonic promotes a three- way completion at the line ending, corresponding to the diamond percept, and the fourth harmonic promotes a four-way completion at both the line endings and at the corners of the illusory figure, corresponding to the illusory square. The Orientational Harmonic simulation shown in the last column of Figure 6 (D) exhibits traces of all three of these illusory phenomena. The Orientational Harmonic model also accounts for the illusory grouping of squares, as shown in Figure 6 (E). At each corner of the square, for example at the top right corner circled in the magnified view in Figure 6 (E), the input signal consists of two orthogonal oriented edges, in this case at six and nine o'clock. The first harmonic response to this input produces a peak at the internal bisector of the two edges, i.e. at 7:30 o'clock, which corresponds to the center of the half-circle containing the greatest oriented signal. The second harmonic would produce a zero response to this input, since its positive and negative peaks are separated by exactly 90 degrees. The third harmonic would attempt to align optimally with the oriented input, with two peaks centered at 5:30 and 9:30 o'clock, leaving a third peak to define an illusory outward projection diagonally at 1:30 o'clock, and the fourth harmonic would align two of its peaks with the six and nine o'clock inputs, leaving two illusory outward projections at twelve and three o'clock. The Orientational Harmonic model therefore predicts illusory grouping lines projecting outward from a square in orthogonal and diagonal directions, as shown in the second column of Figure 6 (E), corresponding to the fourth and third harmonics respectively. These two harmonics however compete with each other by destructive interference, because the diagonal corner projection of the third harmonic corresponds to the negative peak of the fourth harmonic between the orthogonal edge projections. An increase in the strength of the third harmonic would therefore suppress the effect of the fourth harmonic, and vice-versa. This competition is seen in the illusory group ing percept of Figure 6 (E), where the orthogonal alignment of adjacent squares boosts the fourth harmonic signal at each corner, promoting an orthogonal grouping percept, which in turn suppresses the percept of a diagonal grouping. Additional computer simulations (not shown here) reveal that the removal of alternate squares from this figure generates an emergent diagonal grouping percept, which in turn suppresses the perception of an orthogonal grouping. Finally, Figure 6 (F) demonstrates the size/spacing constraint discussed by Zucker, [16] whereby a closer vertical spacing of dots suppresses an equally valid horizontal grouping percept, due to destructive interference between the second and fourth harmonics, because the horizontal grouping lines of the fourth harmonic at three and nine o'clock are suppressed by the negative peaks of the second harmonic response at those same orientations. Lehar [12] shows that removal of alternate rows of dots in this figure restores the horizontal grouping by disinhibition, at the expense of the vertical grouping, even though the horizontal dot spacing remains unchanged. The distance-dependant grouping phenomena seen in Figure 4 (B) and (C) are also explained by harmonic interactions, and have been reproduced in computer simulations [12]. None of the visual phenomena presented in Figure 4 and their replication in computer simulations provide conclusive proof of the Orientational Harmonic model. The fact that this model explains all of these diverse phenomena with a single simple mechanism however makes a strong case for Orientational Harmonics as a mechanism in visual perception. A number of the phenomena shown here have been addressed individually by different models, but no model has yet even attempted to account for such a diversity of phenomena with a single model. The phenomenon of colinear boundary completion is the most straightforward effect, and has been modeled for example by Grossberg's Boundary Contour System (BCS), [6] Walter's Rho Space, [13] and Zucker's curvature operators [15]. All of these models have as their central mechanism some variation of the colinear edge detector cell, which responds to a colinear (or cocircular) arrangement of local edge responses of oriented simple cells, in the manner of the Directed Diffusion model. Some of these models have incorporated some kind of cooperation or competition between orthogonal orientations at each spatial location in order to account for orthogonal end- cut effects as seen in the Ehrenstein illusion of Figure 4 (E), but this kind of model can never account for the generalized vertex completion phenomena seen in Figure 4 (A), (B), and (C). In The original BCS paper [6] Grossberg proposed that the cooperative cell with bipolar receptive fields might also occur in variations with angles other than 180 degrees between the two lobes- for example "L" vertex and "V" vertex detectors with 90 degrees and 30 degrees between the two lobes would account for right angled and acute angled completion. Other cells with three or more lobed receptive fields might account for completion across "T" and "X" vertices. The requirement to have a specialized cell for every vertex configuration however leads to a combinatorial problem, as each specific cell type would have to exist at every orientation at every spatial location in the cortex, and appropriate cooperative and competitive interactions defined between different feature cells. This line of reasoning was never elaborated into a complete theory. A similar combinatorial problem is encountered in Zucker's cocircularity detector cells [15], where "cooperative" cells specialized for edges of every curvature must be replicated at every orientation and every spatial location in the model. This model would suffer further combinatorial problems when extended to vertices composed of more (and less) than two edges. Wilson and Richards [14] present psychophysical evidence that colinear completion gives way abruptly to vertex completion at a particular curvature, which they proposed was evidence for two different mechanisms of curve detection. The same phenomenon was discussed by Kanizsa [10], who illustrated the effect with illusory curves composed of lines of dots, showing that the colinear grouping percept gives way to a sharp vertex grouping at about the same curvature observed by Wilson & Richards. Lehar [12] shows that this effect can be explained by a transition from the second to the third harmonic in the Orientational Harmonic model.

Apologies for the poor quality presentation of the simulation results- here are some of those same results rendered more faithfully.

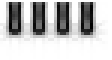
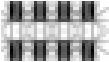
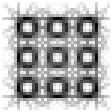
Return to argument

Return to Steve Lehar