
(Footnote: Kind thanks to Eli Peli and the Schepens Eye Research Institute for help and support.)
Home page: .//
email: slehar@cns.bu.edu
Submitted to Behavioral & Brain Sciences September 1999
First revision submitted April 2000
Second revision submitted September 2001
Third revision submitted June 2002
Accepted for publication September 2002
Peer commentaries received February 2003
Response to commentaries submitted March 2003
Response second revision submitted April 2003
Copy-edited article submitted July 2003
Copy-edited response to commentators submitted September 2003
Final paper published volume 26, number 4, pp 375-444, March 2004
Summary of whole review process
Open peer commentaries
Author's response to commentaries
Abstract: 97, 226
Main text: 28698
References: 2293
Entire text: 34946
The subjective experience of visual perception is of a world composed of solid volumes, bounded by colored surfaces, embedded in a spatial void. These properties are difficult to relate to our neurophysiological understanding of the visual cortex. I propose therefore a perceptual modeling approach, to model the information manifest in the subjective experience of perception, as opposed to the neurophysiological mechanism by which that experience is supposedly subserved. A Gestalt Bubble model is presented to demonstrate how the dimensions of conscious experience can be expressed in a quantitative model of the perceptual experience that exhibits Gestalt properties.
A serious crisis is identified in theories of neurocomputation marked by a persistent disparity between the phenomenological or experiential account of visual perception and the neurophysiological level of description of the visual system. In particular conventional concepts of neural processing offer no explanation for the holistic global aspects of perception identified by Gestalt theory. The problem is paradigmatic, and can be traced to contemporary concepts of the functional role of the neural cell, known as the Neuron Doctrine. In the absence of an alternative neurophysiologically plausible model, I propose a perceptual modeling approach, i.e. to model the percept as experienced subjectively, rather than the objective neurophysiological state of the visual system that supposedly subserves that experience. A Gestalt Bubble model is presented to demonstrate how the elusive Gestalt principles of emergence, reification, and invariance, can be expressed in a quantitative model of the subjective experience of visual consciousness. That model in turn reveals a unique computational strategy underlying visual processing, which is unlike any algorithm devised by man, and certainly unlike the atomistic feed-forward model of neurocomputation offered by the Neuron Doctrine paradigm. The perceptual modeling approach reveals the primary function of perception as that of generating a fully spatial virtual-reality replica of the external world in an internal representation. The common objections to this "picture-in-the-head" concept of perceptual representation are shown to be ill founded.
Contemporary neuropscience finds itself in a state of serious crisis. For the deeper we probe into the workings of the brain, the farther we seem to get from the ultimate goal of providing a neurophysiological account of the mechanism of conscious experience. Nowhere is this impasse more evident than in the study of visual perception, where the apparently clear and promising trail discovered by Hubel and Wiesel leading up the hierarchy of feature detection from primary to secondary and to higher cortical areas, seems to have reached a theoretical dead-end. Besides the troublesome issues of the noisy stochastic nature of the neural signal, and the very broad tuning of the single cell as a feature detector, the notion of visual processing as a hierarchy of feature detectors seems to suggest some kind of "grandmother cell" model in which the activation of a single cell or a group of cells represents the presence of a particular type of object in the visual field. However it is not at all clear how such a featural description of the visual scene could even be usefully employed in practical interaction with the world. Alternative paradigms of neural representation have been proposed, including the suggestion that synchronous oscillations play a role in perceptual representation, although these theories are not yet specified sufficiently to know exactly how they address the issue of perceptual representation. But the most serious indictment of contemporary neurophysiological theories is that they offer no hint of an explanation for the subjective experience of visual consciousness. For visual experience is more than just an abstract recognition of the features present in the visual field, but those features are vividly experienced as solid three-dimensional objects, bounded by colored surfaces, embedded in a spatial void. There are a number of enigmatic properties of this world of experience identified decades ago by Gestalt theory, suggestive of a holistic emergent computational strategy whose operational principles remain a mystery.
The problem in modern neuroscience is a paradigmatic one, that can be traced to its central concept of neural processing. According to the Neuron Doctrine, neurons behave as quasi-independent processors separated by relatively slow chemical synapses, with strictly segregated input and output functions through the dendrites and axon respectively. It is hard to imagine how such an assembly of independent processors could account for the holistic emergent properties of perception identified by Gestalt theory. In fact the reason why these Gestalt aspects of perception have been largely ignored in recent decades is exactly because they are so difficult to express in terms of the Neuron Doctrine paradigm. More recent proposals that implicate synchronous oscillations as the neurophysiological basis of conscious experience (Crick & Koch 1990, Crick 1994, Eckhorn et al. 1988, Llinas et al. 1994, Singer 1999, Singer & Gray 1995) seem to suggest some kind of holistic global process that appears to be more consistent with Gestalt principles, although it is hard to see how this paradigm, at least as currently conceived, can account for the solid three-dimensional nature of subjective experience. The persistent disparity between the neurophysiological and phenomenal levels of description suggests that either the subjective experience of visual consciousness is somehow illusory, or that the state of our understanding of neural representation is far more embryonic than is generally recognized.
Pessoa et al. (1998) make the case for denying the primacy of conscious experience. They argue that although the subjective experience of filling-in phenomena is sometimes accompanied by some neurophysiological correlate, that such an isomorphism between experience and neurophysiology is not logically necessary, but is merely an empirical issue, for, they claim, subjective experiences can occur in the absence of a strictly isomorphic correlate. They argue that although the subjective experience of visual consciousness appears as a "picture" or three-dimensional model of a surrounding world, this does not mean that the information manifest in that experience is necessarily explicitly encoded in the brain. That consciousness is an illusion based on a far more compressed or abbreviated representation, in which percepts such as that of a filled-in colored surface can be explained neurophysiologically by "ignoring an absence" rather than by an explicit point-for-point mapping of the perceived surface in the brain.
In fact, nothing could be farther from the truth. For to propose that the subjective experience of perception can be more enriched and explicit than the corresponding neurophysiological state flies in the face of the materialistic basis of modern neuroscience. The modern view is that mind and brain are different aspects of the same physical mechanism. In other words, every perceptual experience, whether a simple percept such as a filled-in surface, or a complex percept of a whole scene, has two essential aspects; the subjective experience of the percept, and the objective neurophysiological state of the brain that is responsible for that subjective experience. Like the two faces of a coin, these very different entities can be identified as merely different manifestations of the same underlying structure, viewed from the internal first-person, v.s. the external third-person perspectives. The dual nature of a percept is analogous to the representation of data in a digital computer, where a pattern of voltages present in a particular memory register can represent some meaningful information, either a numerical value, or a brightness value in an image, or a character of text, etc. when viewed from inside the appropriate software environment, while when viewed in external physical terms that same data takes the form of voltages or currents in particular parts of the machine. However whatever form is selected for encoding data in the computer, the information content of that data cannot possibly be of higher dimensionality than the information explicitly expressed in the physical state of the machine. The same principle must also hold in perceptual experience, as proposed by Müller (1896), in the psychophysical postulate. Müller argued that since the subjective experience of perception is encoded in some neurophysiological state, the information encoded in that conscious experience cannot possibly be any greater than the information encoded in the corresponding neurophysiological state.While we cannot observe phenomenologically the physical medium by which perceptual information is encoded in the brain, we can observe the information encoded in that medium, expressed in terms of the variables of subjective experience. It follows therefore that it should be possible by direct phenomenological observation to determine the dimensions of conscious experience, and thereby to infer the dimensions of the information encoded neurophysiologically in the brain.
The "bottom-up" approach that works upwards from the properties of the individual neuron, and the "top-down" approach that works downwards from the subjective experience of perception are equally valid and complementary approaches to the investigation of the visual mechanism. Eventually these opposite approaches to the problem must meet somewhere in the middle. However to date, the gap between them remains as large as it ever was. Both approaches are essential to the investigation of biological vision, because each approach offers a view of the problem from its own unique perspective. The disparity between these two views of the visual representation can help focus on exactly those properties which are prominently absent from the conventional neural network view of visual processing.
There is a central philosophical issue that underlies discussions of phenomenal experience as seen for example in the distinction between the Gestaltist and the Gibsonian view of perception. That is the epistemological question of whether the world we see around us is the real world itself, or merely an internal perceptual copy of that world generated by neural processes in our brain. In other words this is the question of direct realism, also known as naive realism, as opposed to indirect realism, or representationalism. To take a concrete example, consider the vivid spatial experience of this paper that you hold in your hands. The question is whether the rich spatial structure of this experience before you is the physical paper itself, or whether it is an internal data structure or pattern of activation within your physical brain. Although this issue is not much discussed in contemporary psychology, it is an old debate that has resurfaced several times in psychology, but the continued failure to reach consensus on this issue continues to bedevil the debate on the functional role of sensory processing. The reason for the continued confusion is that both direct and indirect realism are frankly incredible, although each is incredible for different reasons.
The direct realist view is incredible because it suggests that we can have experience of objects out in the world directly, beyond the sensory surface, as if bypassing the chain of sensory processing. For example if light from this paper is transduced by your retina into a neural signal which is transmitted from your eye to your brain, then the very first aspect of the paper that you can possibly experience is the information at the retinal surface, or the perceptual representation downstream of it in your brain. The physical paper itself lies beyond the sensory surface and therefore must be beyond your direct experience. But the perceptual experience of the page stubbornly appears out in the world itself instead of in your brain, in apparent violation of everything we know about the causal chain of vision. Gibson explicitly defended the notion of direct perception, and spoke as if perceptual processing occurs somehow out in the world itself rather than as a computation in the brain based on sensory input (Gibson 1972 p. 217 & 239). Significantly, Gibson refused to discuss sensory processing at all, and even denied that the retina records anything like a visual image that is sent to the brain. This leaves the status of the sensory organs in a peculiar kind of limbo, for if the brain does not process sensory input to produce an internal image of the world, what is the purpose of all that computational wetware? Another embarrassment for direct perception is the phenomenon of visual illusions, which are observed out in the world itself, and yet they cannot possibly be in the world, for they are the result of perceptual processing that must occur within the brain. With characteristic aplomb, Gibson simply denied that illusions are illusory at all, although it is not clear exactly what he could possibly have meant by that. Modern proponents of Gibson's theories usually take care to disclaim his most radical views (Bruce & Green 1987 p. 190, 203-204, Pessoa et al. 1998, O'Regan 1992 p. 473) but they present no viable alternative explanation to account for our experience of the world beyond the sensory surface.
The difficulty with the concept of direct perception is most clearly seen when considering how an artificial vision system could be endowed with such external perception. Although a sensor may record an external quantity in an internal register or variable in a computer, from the internal perspective of the software running on that computer, only the internal value of that variable can be "seen", or can possibly influence the operation of that software. In exactly analogous manner the pattern of electrochemical activity that corresponds to our conscious experience can take a form that reflects the properties of external objects, but our consciousness is necessarily confined to the experience of those internal effigies of external objects, rather than of external objects themselves. Unless the principle of direct perception can be demonstrated in a simple artificial sensory system, this explanation remains as mysterious as the property of consciousness it is supposed to explain.
The indirect realist view is also incredible, for it suggests that the solid stable structure of the world that we perceive to surround us is merely a pattern of energy in the physical brain, i.e. that the world that appears to be external to our head is actually inside our head. This could only mean that the head we have come to know as our own is not our true physical head, but is merely a miniature perceptual copy of our head inside a perceptual copy of the world, all of which is completely contained within our true physical skull. Stated from the internal phenomenal perspective, out beyond the farthest things you can perceive in all directions, i.e. above the dome of the sky and below the earth under your feet, or beyond the walls, floor, and ceiling of the room you perceive around you, beyond those perceived surfaces is the inner surface of your true physical skull encompassing all that you perceive, and beyond that skull is an unimaginably immense external world, of which the world you see around you is merely a miniature virtual-reality replica. The external world and its phenomenal replica cannot be spatially superimposed, for one is inside your physical head, and the other is outside. Therefore the vivid spatial structure of this page that you perceive here in your hands is itself a pattern of activation within your physical brain, and the real paper of which it is a copy it out beyond your direct experience. I have found a curious dichotomy in the response of colleagues in discussions on this issue. For many people will agree with the statement that everything you perceive is in some sense inside your head, and in fact they often complain that this is so obvious it need hardly be stated. However when that statement is turned around to say that out beyond everything you perceive is your physical skull, to this they object most vehemently as being absurd. And yet the two statements are logically identical, so how can one appear trivially obvious while the other seems patently absurd? This demostrates the value of this particular mental image, for it helps to smoke out any residual naive realism that may remain hidden in our philosophy. For although this statement can only be true in a topological, rather than a strict topographical sense, this insight emphasizes the indisputable fact that no aspect of the external world can possibly appear in consciousness except by being represented explicitly in the brain. The existential vertigo occasioned by this mental image is so disorienting that only a handful of researchers have seriously entertained this notion or pursued its implications to its logical conclusion. (Kant 1781/1991, Koffka 1935, Köhler 1971 p. 125, Russell 1927 pp 137-143, Smythies 1989, 1994, Harrison 1989, Hoffman 1998, Lehar 2003)
Another reason why the indirect realist view is incredible is that the observed properties of the world of experience when viewed from the indirect realist perspective are difficult to resolve with contemporary concepts of neurocomputation. For the world we perceive around us appears as a solid spatial structure that maintains its structural integrity as we turn around and move about in the world. Perceived objects within that world maintain their structural integrity and recognized identity as they rotate, translate, and scale by perspective in their motions through the world. These properties of the conscious experience fly in the face of everything we know about neurophysiology, for they suggest some kind of three-dimensional imaging mechanism in the brain, capable of generating three-dimensional volumetric percepts of the degree of detail and complexity observed in the world around us. No plausible mechanism has ever been identified neurophysiologically that exhibits this incredible property. The properties of the phenomenal world are therefore inconsistent with contemporary concepts of neural processing, which is exactly why these properties have been so long ignored.
The perceived incredibility of both direct and indirect realism has led many over the centuries to propose that conscious experience is located neither in the physical brain, nor in the external world, but in some other separate space that bears no spatial relation to the physical space known to science. These theories fall somewhere between direct and indirect perception, because they claim that phenomenal experience is neither in the head, nor is it out in the world. The original formulation of this thesis was Cartesian dualism—the traditional religious or spiritual view that mind exists in a separate realm which is inaccessible to science. Our inability to detect spiritual entities is not due to any limitations of our detector technology, but to the fact that spiritual entities are impossible in principle to detect by physical means. Cartesian dualism is a minority position in contemporary philosophy, at least as a scientific theory of mind, and for very good reason. The chief objection to this kind of dualism is Occam's razor: it is more parsimonious to posit a single universe with one set of physical laws, rather than two radically dissimilar parallel universes composed of dissimilar substance and following dissimilar laws, making tenuous contact with each other nowhere else but within a living conscious brain. But if mind and matter come into causal contact, as they clearly do in both sensory and motor function, then surely they must be different parts of one and the same physical universe. But there is another, still more serious objection to Cartesian dualism than the issue of parsimony. Since the experiential, or spiritual component of the theory is in principle inaccessible to science, that portion of the theory can be neither confirmed nor refuted. This places the spiritual component of Cartesian dualism beyond the bounds of science, and firmly in the realm of religious belief.
A more sophisticated half-way epistemology is seen in the philosophy of critical realism (Sellars 1916, Russell 1921, Broad 1925, Drake et al. 1920). While the critical realists avoid religious explanations involving God or spirits, their concept of conscious experience nevertheless preserves some of the mystery of Cartesian dualism. Critical realists acknowledge that perception is not direct, but is mediated by an intermediate representational entity which they call sense-data. However critical realists insist that the sense-data are neither physical nor mental, but "particular existents of a peculiar kind; they are not physical, ... and there is no reason to suppose that they are either states of mind or existentially mind-dependent. In having spatial characteristics ... they resemble physical objects ... but in their privacy and their dependence on the body ... of the observer they are more like mental states." (Broad 1925, p. 181) As with the spirit world of the Cartesian view, sense data and the space in which they are observed are not just difficult to detect, but they are in principle beyond scientific scrutiny. There is some debate among critical realists over the ontology of conscious experience. In a book on critical realism by a consortium of authors (Drake et al. 1920) Lovejoy, Pratt, and Sellars claim that the sensa are completely "the character of the mental existent ... although its existence is not given", while Drake, Rogers, Santayana, and Strong agree that the data are characteristic of the apprehended object, although "the datum is, qua datum, a mere essence, an inputed but not necessarily actual existent. It may or may not have existence." (p. 20-21 footnote). So the critical realists solved the epistemological problem by defining a unique kind of existent that is experienced, but that does not, or may not actually exist. This is a peculiar inversion of the true epistemological situation, because in fact sense data, or the raw material of conscious experience, are the only thing which we can know with any real certainty to actually exist. All else, including the entire physical world known to science, is informed conjecture based on that experience.
A more modern reformulation of this muddled epistemology is seen in Davidson's anomalous monist thesis (Davidson 1970). Davidson suggests that the mental domain, on account of its essential anomalousness and normativity cannot be the object of serious scientific investigation, because the mental is on a wholly different plane from the physical. This argument sounds like the metaphysical dualism of Descartes that disconnects mind from brain entirely, except that Davidson qualifies his theory with the monistic proviso that every mental event is connected with specific physical events (in the brain), although there are no laws connecting mental kinds with physical kinds, and this presumably rescues the thesis from metaphysical dualism. Kim (1998) points out however that this is a negative thesis, for it tells us only how the mental is not related to the physical, it says nothing about how they are related. As such this is more an article of faith rather than a real theory of any sort, and in the context of the history of the epistemological debate this can be seen as a last desperate attempt to rescue naïve realism from its own logical contradictions. This kind of physicalism has been appropriately dubbed `token physicalism', for it is indeed a token admission of the undeniable link between mind and the physical brain, without admitting to any of its very significant implications.
In order to rationalize this view of the mind-brain relation Davidson introduces the peculiar notion of supervenience, a one-way asymmetrical relation between mind and brain that makes mind dependent on the brain, but that forever closes the possibility of phenomenological observation of brain states. As in the case of Cartesian dualism, there are two key objections to this argument. In the first place the disconnection between the experiential mind and the physical brain is itself merely a hypothesis, whose truth remains to be demostrated. It is at least equally likely prima facie that the mind does not supervene on the brain, but that mind is identically equal to the functioning of the physical brain. In fact, this is by far the more parsimonious explanation, because it invokes a single explanans, the physical brain, to account for the properties of both mind and brain. After all, physical damage to the brain can result in profound changes in the mind—not just the information content of the mind, nor just observed behavior, but brain damage can produce profound changes in the experiential, or "what it is like" aspect of conscious experience. The simplest explanation therefore is that consciousness is a physical process taking place in the physical brain, which is why it is altered by physical changes to the physical brain.
But the problem of supervenience is more serious than just the argument of parsimony. For if the properties of mind were indeed disconnected from the properties of the physical brain, this would leave the mental domain completely disconnected from the world of reality known to science, what Feigl (1958) has called a "nomological dangler." For if the properties of mind are not determined by the properties of the physical brain, what is it that determines the properties of the mind? For example phenomenal color experience has been shown to be reducible to the three dimensions of hue, intensity, and saturation. Physical light is not restricted to these three dimensions; the spectrum of a typical sample of colored light contains a separate and distinct magnitude for every spectral frequency of the light, an essentially infinite-dimensional space that is immeasurably greater in information content than the three dimensions of phenomenal color experience. In answer to Koffka's (1935) classical question—"Why do things look as they do?" the answer is clearly not "Because they are what they are." That answer that is clearly false in the case of color perception, as well as in the case of visual illusions, not to mention dreams and hallucinations. We now know that the dimensionality of color experience relates directly to the physiology of color vision, i.e. it relates to the fact that there are three different cone types in the human retina, and to the opponent color process representation in the visual cortex. The dimensions of color experience therefore are not totally disconnected from the properties of the physical brain as suggested by Davidson, but in fact phenomenal color experience tells us something very specific about the properties of the representation of color in the physical brain. And the same argument holds for spatial vision, for there are a number of prominent distortions of phenomenal space that clearly indicate that phenomenal space is ontologically distinct from the physical space known to science, as will be discussed later.
Daniel Dennett (1991) promotes a similar half-way epistemology by drawing a distinction between the neural vehicles of mental representation, and the phenomenal contents of those vehicles. Dennett opens the epistemological crack by claiming that the phenomenal contents do not necessarily bear any similarity whatsoever to the neural vehicles by which they are encoded in the brain. This actually goes beyond Davidson's supervenience, because according to Davidson, mental events that are distinct phenomenally, must be distinct neurophysiologically also. This is tantamount to saying that the dimensions of conscious experience cannot be any greater than the dimensions of the corresponding neurophysiological state. Dennett effectively removes this limitation by suggesting that even the dimensionality of the phenomenal contents need not match that of the neural vehicles. And into that epistemological crack, Dennett slips the entire world of conscious experience like a magical disappearing act, where it is experienced, but does not actually exist. But by the very fact that conscious experience, as conceived by Dennett, is in principle undetectable by scientific means, this concept of consciousness becomes a religious rather than a scientific hypothesis, whose existence can be neither confirmed nor refuted by scientific means. In fact Dennett even suggests that there is actually no such thing as consciousness per se, and that belief in consciousness is akin to belief in some kind of mythical nonexistent deity (Dennett 1981). This argument of course is only intelligable from a naïve realist perspective, by which the sense-data of conscious experience, so plainly manifest to one and all, are mis-identified as the external world itself, rather than as something going on in the physical brain.
Another modern theorist, Max Velmans (1990), revives an ancient notion of perception as something projecting out of the head into the world, as proposed by Empedocles and promoted by Malebranche. But Velmans refines this ancient notion with the critical realist proviso that nothing physical gets actually projected from the head, the only thing that is projected is conscious experience, a subjective quality that is undetectable externally by scientific means. But again, as with critical realism, the problem with this notion is that the sense data that are experienced to exist, do not exist in any true physical sense, and therefore the projected entity in Velman's theory is a spiritual entity to be believed in, (for those who are so inclined) rather than anything knowable by, or demonstrable to science. Velmans draws the analogy of a videotape recording, that carries the information of a dynamic pictorial scene expressed in a highly compressed and non-spatial representation, as patterns of magnetic fields on the tape. There is no resemblance or isomorphism between the magnetic tape and the images that it encodes, except for its information content. However the only reason that the videotape even represents a visual scene is because of the existence of video technology that is capable of reading that magnetic information from the tape and sweeping it out as a spatial image on a video monitor or television screen, where each pixel appears in its proper place in the image. If that equipment did not exist, then there would be no images as such on the videotape. But if video technology is to serve as an analogy for spatial representation in the brain, the key question is whether the brain encodes that pictorial information exclusively in the abstract compressed form like the magnetic patterns on the tape, or whether the brain reads those compressed signals and projects them as an actual spatial image somewhere in the brain like a television monitor, whenever we have a visuospatial experience. For if it is the former, then sense data are experienced, but do not actually exist as a scientific entity, so the spatial image we see is a complete illusion, which again, is an inversion of the true epistemology. If it is the latter, then that means that there are actual "pictures in the head", a notion that Velmans emphatically rejects.
In fact the only epistemology that is consistent with the modern materialistic world view is an identity theory (Russell 1927, Feigl 1958) whereby mind is identically equal to physical patterns of energy in the physical brain. To claim otherwise is to relegate the elaborate structure of conscious experience to a mystical state beyond the bounds of science. The dimensions of conscious experience, such as phenomenal color and phenomenal space, are a direct manifestation of certain physical states of our physical brain. The only correct answer to Koffka's question is that things appear as they do because that is the way the world is represented in the neurophysiological mechanism of our physical brain. The world of conscious experience therefore is in principle accessible to scientific scrutiny after all, both internally through introspection, and externally through neurophysiological recording. And introspection is as valid a method of investigation as is neurophysiology, just as in the case of color experience. Of course the mind can be expected to appear quite different from these two different perspectives, just as the data in a computer memory chip appears quite different when examined internally by data access, as opposed to externally by electrical probes. But the one quantity that is preserved across the mind/brain barrier is information content, and therefore that quantity can help identify the neurophysiological mechanism or principle in the brain whose dimensionality, or information content, matches the observed dimensions of conscious experience.
We are left therefore with a choice between three alternatives, each of which appears to be absolutely incredible. Contemporary neuroscience seems to take something of an equivocal position on this issue, recognizing the epistemological limitations of the direct realist view and of the projection hypothesis, while being unable to account for the incredible properties suggested by the indirect realist view. However one of these three alternatives simply must be true, to the exclusion of the other two. And the issue is by no means inconsequential, for these opposing views suggest very different ideas of the function of visual processing, or what all that neural wetware is supposed to actually do. Therefore it is of central importance for psychology to address this issue head-on, and to determine which of these competing hypotheses reflect the truth of visual processing. For until this most central issue is resolved definitively, psychology is condemned to remain in what Kuhn (1970) calls a pre-paradigmatic state, with different camps arguing at cross-purposes due to a lack of consensus on the foundational assumptions and methodoligies of the science. For psychology is, after all, the science of the psyche, i.e. subjective side of the mind/brain barrier, and neurophysiology only enters into the picture to provide a physical substrate for mind. Therefore it is of vital importance to reach a consensus on the nature of the explanandum of psychology before we can attempt an explanans. In particular, we must decide whether the vivid spatial structure of the surrounding world of visual experience is an integral part of the psyche and thus within the explanandum of psychology, or whether it is the external world itself, as it appears to be naively, and thus in the province of physics rather than of psychology.
The problem with the direct realist view is of an epistemological nature, and is therefore a more fundamental objection, for direct realism as defended by Gibson is nothing short of magical, that we can see the world out beyond the sensory surface. The projection theory has a similar epistemological problem, and is equally magical and mysterious, suggesting that neural processes in our brain are somehow also out in the world. Both of these paradigms have difficulty with phenomena of dreams and hallucinations (Revonsuo 1995), which present the same kind of phenomenal experience as spatial vision, except independently of the external world in which that perception is supposed to occur in normal vision. It is the implicit or explicit acceptance of this naive concept of perception that has led many to conclude that consciousness is deeply mysterious and forever beyond human comprehension. For example Searle (1992 p. 96) contends that consciousness is impossible to observe, for when we attempt to observe consciousness we see nothing but whatever it is that we are conscious of; that there is no distinction between the observation and the thing observed.
The problem with the indirect realist view on the other hand is more of a technological or computational limitation, for we cannot imagine how contemporary concepts of neurocomputation, or even artificial computation for that matter, can account for the properties of perception as observed in visual consciousness. It is clear however that the most fundamental principles of neural computation and representation remain to be discovered, and therefore we cannot allow our currently limited notions of neurocomputation to constrain our observations of the nature of visual consciousness. The phenomena of dreams and hallucinations clearly demonstrate that the brain is capable of generating vivid spatial percepts of a surrounding world independent of that external world, and that capacity must be a property of the physical mechanism of the brain. Normal conscious perception can therefore be characterized as a guided hallucination (Revonsuo 1995), which is as much a matter of active construction as it is of passive detection. If we accept the truth of indirect realism, this immediately disposes of at least one mysterious or miraculous component of consciousness, which is its unobservability. For in that case consciousness is indeed observable, contrary to Searle's contention, because the objects of experience are first and foremost the product or "output" of consciousness, and only in secondary fashion are they also representative of objects in the external world. Searle's difficulty in observing consciousness is analogous to saying that you cannot see the moving patterns of glowing phosphor on your television screen, all you see is the ball game that is showing on that screen. The indirect realist view of television is that what you are seeing is first and foremost glowing phosphor patterns on a glass screen, and only in secondary fashion are those moving images also representative of the remote ball game.
The choice therefore is that either we accept a magical mysterious account of perception and consciousness that seems impossible in principle to implement in any artificial vision system, or we have to face the seemingly incredible truth that the world we perceive around us is indeed an internal data structure within our physical brain (Lehar 2003). The principal focus of neurophysiology should now be to identify the operational principles behind the three-dimensional volumetric imaging mechanism in the brain, that is responsible for the generation of the solid stable world of visual experience that we observe to surround us in conscious experience.
The computational modeling of perceptual processes is a formidable undertaking. But the problem is exacerbated by the fact that a neural network model of perception attempts to model two entities simultaneously, the subjective experience of perception, and the neurophysiological mechanism by which that experience is generated in the brain. The chief problem with this approach is that our knowledge of neurophysiological principles is known to be incomplete. We do not understand the computational functionality of even the simplest neural systems. For example, the lowly house fly, with its tiny pinpoint of a brain, seems to thumb its nose at our lofty algorithms and complex computational models, as it dodges effortlessly between the tangled branches of a shrub in dappled sunlight, compensating for gusty cross-winds to avoid colliding with the branches. This remarkable performance by this lowly creature far exceeds the performance of our most powerful computer algorithms, and our most sophisticated neural network models of human perception. In fact, the "dirty little secret" of neuroscience, as Searle ( 1997, p. 198) calls it, is that we have no idea what the correct level of analysis of the brain should be, because there is no universally accepted theory of how the brain actually codes perceptual or experiential information. The epistemological question highlights this uncertainty, for it shows that there is not even concensus on whether the world of conscious experience is even explicitly represented in the brain at all, the majority view being, apparently, that it is not. Palmer (1999) goes even farther to say: "to this writer's knowledge, no one has ever suggested any theory that the scientific community regards as giving even a remotely plausible causal account of how experience arises from neural events." Without this key piece of knowledge, how can we even begin to model the computational processes of perception in neurophysiological terms?
One approach is to begin with the neurophysiology of the brain, and attempt to discover what it is computing at the local level of the individual neuron, the elemental building block of the nervous system. The fruit of this branch of investigation is neural network theory. But it is unclear whether neural network theory offers an adequate characterization of the actual processing going on in the brain, or whether it is simply asking too much of simple integrate-and-fire elements, no matter how cleverly connected in patterns of synaptic connections, to provide anything like an adequate account of the observed properties of conscious experience. Churchland (1984) argues in the affirmative, that we do have enough knowledge of the principles of neurocomputation to begin to propose realistic models of perceptual processing. Palmer (1992) and Opie (1999) present dynamic neural network models of Gestalt phenomena, such as the perceptual grouping of triangles, showing how the dynamics of perceptual phenomena can be modeled by a dynamic neural network model. But those models are proposed in the abstract, presenting general principles rather than complete and detailed models of specific perceptual phenomena expressed as sense-data. For example Palmer (1992) discusses the perceptual experience of an equilateral triangle, which is perceived as an arrow that is pointing in one of three directions. Palmer models this perceptual competition as a competition between three dynamic neural network nodes in a mutually inhibitory relationship, resulting in a "winner-take-all" behavior. Although this model is compelling as a demonstration of Gestalt principles in a neural network model, Palmer leaves out the most difficult part of the problem, which is not just the competition between three alternative percepts, but the perceptual representation of the percept itself. For the perceptual experience of a triangle cannot be reduced to just three phenomenal values, but is observed as a fully reified triangular structure that spans a specific portion of perceived space. This "sense-data" component of the phenomenal experience is very much more difficult to account for in neural network terms.
There have been a number of attempts in recent decades to quantify the sense-data of visual consciousness in computational models (see Lesher 1995 for a review). For example Zucker et al. (1988) present a model of curve completion that accounts for the emergent nature of perceptual processing by incorporating a feedback loop in which local feature detectors tuned to detect oriented edges feed up to global curvature detector cells, and those cells in turn feed back down to the local edge level to fill in missing pieces of the global curve. A similar bottom-up/top-down feedback is found in Grossberg & Mingolla's (1985) visual model to account for boundary completion in illusory figures like the Kanizsa square by generating an explicit line of neural activation along the illusory contour. An extension of that model (Grossberg & Todoroviçz 1988) accounts for the filling-in of the surface brightness percept in the Kanizsa figure, with an explicit diffusion of neural activation within the region of the illusory surface.These models have had a significant impact on the discussion of the nature of visual illusions because they highlight the fact that illusory features, like the illusory surface of a Kanizsa figure, are observed as extended image-like data structures, and that therefore a complete model of the phenomenon must also produce a fully reified image-like spatial structure as its output. In fact, Grossberg's concept of visual reification in his Boundary Contour System (Grossberg & Mingolla 1985) and Feature Contour System (Grossberg & Todoroviçz 1988) were the original inspiration behind the perceptual modeling proposed in the present hypothesis.
But while these models finally offer a reasonable account of perceptual experience (in two dimensions), they also demonstrate the profound limitations of a neural network architecture for perceptual representation. Because neural network theory is no different in principle than a template theory, (Lehar 2002) a concept whose limitations are well known. Grossberg & Mingolla (1985) account for collinear illusory contour completion by way of specialized elongated receptive fields, tuned to detect and enhance collinearity. Although this concept works well enough for simple collinear boundary completion (as long as it remains restricted to two dimensions), any attempt to extend this model to higher order perceptual processing runs headlong into a combinatorial explosion in required receptive fields. (Lehar 2002) For example perceptual completion is observed not only for collinear alignments, but can define illusory vertices composed of two, three, or more edges that meet at a vertex. (Lehar 2002) Grossberg himself proposed an extension to his model equipped with "corner detector" receptive fields, (Grossberg 1985), although this line of thought was subsequently quietly abandoned. Because just as with the cells that perform collinear completion, the corner detectors would have to be provided at every location and every orientation across the visual field. And to extend the model to account for "T," "V," "Y" and "X" intersections, specialized receptive fields would have to be provided for each of those features at every location and at every orientation across the visual field. This combinatorial explosion in the required number of specialized receptive fields does not bode well for neural network theory as a general principle of neurocomputation.
But the most serious limitation of Grossberg's approach to perception is that curiously, Grossberg and his colleagues do not extend their logic to the issue of three-dimensional spatial perception. For in going from two dimensions to three, Grossberg no longer advocates explicit spatial filling-in, but instead represents the depth dimension by binocular disparity, using left and right eye image pairs. (Grossberg 1987b, 1990, 1994) Although a stereo pair does encode depth information, it does not do so in a volumetric manner, because it can only encode one depth or disparity value for every (x,y) point on the image. This makes it impossible for Grossberg's model to represent transparency, with multiple depth values at a single (x,y) location, or to represent the experience of empty space between the observer and a visible object, and it precludes the kind of volumetric filling-in required to account, for example, for the three-dimensional version of the Ehrenstein illusion constructed of a set of rods, arranged radially around a circular void. (Ware & Kennedy 1978) The filling-in processes in this illusion take place through the depth dimension, which produces an illusory percept of a glowing disk, hanging in space, as a volumetric spatial structure. If Grossberg's argument for explicit filling-in of the two-dimensional illusions is at all valid, then that argument should apply equally to volumetric filling-in also.
The reason why Grossberg declines to extend his model into the third dimension is neurophysiologically motivated. For although Grossberg's model is a de-facto perceptual model, it is actually presented as a neural network model, i.e. the computational units of the model represent actual neurons in the brain, rather than perceptual entities. And this highlights the problem of perceptual modeling in neural network terms. For whenever there is a conflict between the perceptual phenomenon and our current understanding of neurophysiological principles, then there is a conflict between the neural and the perceptual models of the phenomenon. In this case the percept is clearly volumetric, but the corresponding cortical neurophysiology is assumed to be two-dimensional. Another reason Grossberg is reluctant to extend his model into the third dimension is that even for simple collinear completion, this would require a volumetric block of neural elements, each equipped with elongated receptive fields, and those fields must be replicated at every orientation in three dimensions, at every volumetric location across the entire volume of the perceptual representation, a notion that seems too implausible to contemplate, let alone the idea of "T," "V," "Y" and "X" intersections defined in three dimensions. But until a mapping has been established between the conscious experience and the corresponding neurophysiological state, there is no way to verify whether the model has correctly replicated the psychophysical data. Since these models straddle the mind/brain barrier, they run headlong into the issue which Chalmers has dubbed the "hard problem" of consciousness (Chalmers 1995). Simply stated, even if we were to discover the exact neurophysiological correlates of conscious experience, there will alway remain a final explanatory gap between the physiological and the phenomenal levels of description. For example if the activation of a particular cell in the brain were found to correlate with the experience of red at some point in the visual field, there remains a vivid subjective quality, or quale, to the experience of red which is not in any way identical to any externally observable physical variable such as the electrical activity of a cell. In other words there is a subjective experiential component of perception that can never be captured in a model expressed in objective neurophysiological terms.
Even more problematic for neural models of perception is the question of whether perceptual information is expressed neurophysiologically in explicit or implicit form. For example Dennett (1992) argues that the perceptual experience of a filled-in colored surface is encoded in more abstracted form in the brain, in the manner of an edge image that records only the transitions along image edges. Support for this concept is seen in the retinal ganglion cells, that respond only along spatial or temporal discontinuities in the retinal image, and produce no response within regions of uniform color or brightness. This concept also appears to make sense from an information-theoretic standpoint, for uniform regions of color represent redundant information that can be compressed to a single value, as is the practice in image compression algorithms. These kinds of theoretical difficulties have led many neuroscientists to simply ignore the conscious experience, and to focus instead on the hard evidence of the neurophysiological properties of the brain.
The quantification of conscious experience is not quite as hopeless as it might seem. Nagel (1974) suggests that we set aside temporarily the relation between mind and brain and devise a new method of objective phenomenology, i.e. to quantify the structural features of the subjective experience in objective terms, without committing to any particular neurophysiological theory of perceptual representation. For example if we quantify the experience of vision as a three-dimensional data structure, like a model of volumes and surfaces in a surrounding space to a certain perceptual resolution, this description could be meaningful even to a congenitally blind person, or an alien creature who had never personally experienced the phenomenon of human vision. While this description could never capture everything of that experience, such as the qualia of color experience, it does at least capture the structural characteristics of that subjective experience in an objective form that would be comprehensible to beings incapable of having those experiences. Chalmers (1995) extends this line of reasoning with the observation that the subjective experience and its corresponding neurophysiological state carry the same information content. Chalmers therefore proposes a principle of structural coherence between the structure of phenomenal experience and the structure of objectively reportable awareness, to reflect the central fact that consciousness and physiology do not float free of one another, but cohere in an intimate way. In essence this is a restatement of the Gestalt principle of isomorphism, of which more later. The connecting link between mind and brain therefore is information in information-theoretic terms (Shannon 1948), because the concept of information is defined at a sufficiently high level of abstraction to be independent of any particular physical realization, and yet it is sufficiently specified as to be measurable in any physical system given that the coding scheme is known. A similar argument is made by Clark (1993, p. 50). Chalmers moderates his claim of the principle of structural coherence by stating that it is a hypothesis that is "extremely speculative". However the principle is actually solidly grounded epistemologically because the alternative is untenable. If we accept the fact that the physical states of the brain correlate directly with conscious experience, then the claim that conscious experience contains more explicit information than the physiological state on which it was based, amounts to a kind of dualism that would necessarily involve some kind of non-physical "mind stuff" to encode the excess information observed in experience that is not encoded by the physical state. Some theorists have even proposed a kind of hidden dimension of physical reality to house the unaccounted information in conscious experience (Harrison 1989, Smythies 1994).
The philosophical problems inherent in neural network models of perceptual experience can be avoided by proposing a perceptual modeling approach (Lehar 2003), as opposed to neural modeling, i.e. to model the conscious experience directly, in the subjective variables of perceived color, shape, and motion, rather than in the neurophysiological variables of neural activations or spiking frequencies etc. The variables encoded in the perceptual model therefore correspond to what philosophers call the "sense-data" or primitives of raw conscious experience, except that these variables are not supposed to be the sense-data themselves, they merely represent the value or magnitude of the sense-data that they are defined to represent. In essence this amounts to modeling the information content of subjective experience, which is the quantity that is common between the mind and brain, thus allowing an objectively quantified description of a subjective experience. In fact this approach is exactly the concept behind the description of phenomenal color space in the dimensions of hue, intensity, and saturation, as seen in the CIE chromaticity space. The geometrical dimensions of that space have been tailored to match the properties of the subjective experience of color as measured psychophysically, expressed in terms that are agnostic to any particular neurophysiological theory of color representation. Clark (1993) presents a systematic description of other sensory qualities in quantitative terms, based on this same concept of `objective phenomenology'. The thorny issue of the `hard problem' of consciousness is thus neatly side-stepped, because the perceptual model remains safely on the subjective side of the mind / brain barrier, and therefore the variables expressed in the model refer explicitly to subjective qualia rather than to neurophysiological states of the brain. The problems of explicit v.s. implicit representation are also neatly circumvented, because those issues pertain to the relation between mind and brain, and therefore they do not apply to a model that does not straddle the mind/brain barrier. For example the subjective experience of a Necker cube is of a solid three-dimensional structure, and therefore the perceptual model of that experience should also be an explicit three-dimensional structure. The spontaneous reversals of the Necker cube on the other hand are experienced as a dynamic process, and therefore that should be represented in the perceptual model as a dynamic process, i.e. as a literal reversal of the solid three-dimensional structure. The issue of whether a perceived structure can be encoded neurophysiologically as a process, or whether a perceived process can be encoded as a structure, are therefore irrelevant to the perceptual model, which by definition models a perceived structure as a structure, and a perceived process as a process.
While this is of course only an interim solution, for eventually the neurophysiological basis of conscious experience must also be identified, the perceptual model does offer objective information about the informational content encoded in the physical mechanism of the brain. This is a necessary prerequisite to a search for the neurophysiological basis of conscious experience, for we must clearly circumscribe that which we are to explain, before we can attempt an explanation of it. This approach has served psychology well in the past, particularly in the field of color perception, where the quantification of the dimensions of color experience led directly to great advances in our understanding of the neurophysiology of color vision. The failure to quantify the dimensions of spatial experience has been responsible for decades of futile debate about its neurophysiological correlates. I will show that application of this perceptual modeling approach to the realm of spatial vision opens a wide chasm between phenomenology and contemporary concepts of neurocomputation, and thereby offers a valuable check on theories of perception based principally on neurophysiological concepts.
The Gestalt principle of isomorphism represents a subtle but significant extension to Müller's psychophysical postulate, and Chalmers' principle of structural coherence. For in the case of structured experience, equal dimensionality between the subjective experience and its neurophysiological correlate implies similarity of structure or form. For example the percept of a filled-in colored surface, whether real or illusory, encodes a separate and distinct experience of color at every distinct spatial location within that surface to a particular resolution. Each point of that surface is not experienced in isolation, but in its proper spatial relation to every other point in the perceived surface. In other words the experience is extended in (at least) two dimensions, and therefore the neurophysiological correlate of that experience must also encode (at least) two dimensions of perceptual information. The mapping of phenomenal color space was established by the method of multidimensional scaling (Coren et al. 1994 p. 57) in which color values are ordered in psychophysical studies based on their perceived similarity, to determine which colors are judged to be nearest to each other, or which colors are judged to be between which other colors in phenomenal color space. A similar procedure could just as well be applied to spatial perception to determine the mapping of phenomenal space. If two points in a perceived surface are judged psychophysically to be nearer to each other when they are actually nearer, and farther when they are actually farther, and if other spatial relations such as between-ness etc. are also preserved phenomenally, this provides direct evidence that phenomenal space is mapped in a spatial representation that preserves those spatial relations in the stimulus. The outcome of this proposed experiment is so obvious it need hardly be performed. And yet its implications, that our phenomenal representation of space is spatially mapped, is not often considered in contemporary theories of spatial representation.
The isomorphism required by Gestalt theory is not a strict structural isomorphism, i.e. a literal isomorphism in the physical structure of the representation, but merely a functional isomorphism, i.e. a behavior of the system as if it were physically isomorphic (Köhler 1969, p 92). For the exact geometrical configuration of perceptual storage in the brain cannot be observed phenomenologically any more than the configuration of silicon chips on a memory card can be determined by software examination of the data stored within those chips. Nevertheless the mapping between the stored perceptual image and the corresponding spatial percept must be preserved, as in the case of the digital image also, so that every stored color value is meaningfully related to its rightful place in the spatial percept.
The distinction between structural and functional isomorphism can be clarified with a specific example. Consider the spatial percept of a block resting on a surface depicted schematically in figure 1 A. The information content of this perceptual experience can be captured in a painted cardboard model built explicitly like figure 1 A, i.e. with explicit volumes, bounded by colored surfaces, embedded in a spatial void. Since perceptual resolution is finite, the model should also be considered only to a finite resolution, i.e. the infinite subdivision of the continuous space of the actual model world is not considered part of the model, which can only validly represent subdivision of space to the resolution limit of perception. The same perceptual information can also be captured in quantized or digital form in a volumetric or "voxel" (volume-pixel) image in which each voxel represents a finite volume of the corresponding perceptual experience, as long as the resolution of this representation matches the spatial resolution of the percept itself, i.e. the size of the voxels should match the smallest perceivable feature in the corresponding spatial percept. Both the painted cardboard model, and its quantized voxel equivalent, are structurally or topographically isomorphic with the corresponding percept, i.e. they have the same information content as the spatial percept that they represent.

Consider now the flattened representation depicted in figure 1 B, which is identical to the model in figure 1 A except that in this case the depth dimension is compressed relative to the other two, like a bas-relief sculpture. If the defined scale of the model, i.e. the length in the representation relative to the length that it represents, is also correspondingly compressed, as suggested by the compressed grid lines in the figure, then this model is also isomorphic with the perceptual experience of figure 1 A. In other words the flattening of the depth dimension is not really registered in the model, because the perceived cube spans the same number of grid lines in figure 1 B (in all three dimensions) as it does in figure 1 A, and therefore this flattened model encodes a non-flattened perceptual experience. However this model is now no longer structurally isomorphic with the original perceptual experience, although it remains topologically isomorphic, preserving neighborhood relations, as well as between-ness etc. In a mathematical system with infinite resolution this model would encode the same information as the one in figure 1 A. However in a real physical representation there is always some limit to the resolution of the system, or how much information can be stored in each unit distance in the model itself. In a representational system with finite resolution therefore, the depth information in figure 1 B would necessarily be encoded at a lower resolution than that in the other two dimensions. If our own perceptual apparatus employed this kind of representation, this flattening would not be experienced directly; the only manifestation of the flattening of the representation would be a reduction in the resolution of perceived depth, relative to the other two dimensions, i.e. it would be more difficult to distinguish differences of perceived depth than differences of perceived height and width.
Consider now the warped model depicted in figure 1 C, which is like the flattened model of figure 1 B with a wavy distortion applied, as if warped like the gyri and sulci of the cortical surface. This warped representation is also isomorphic with the perceptual experience it represents, i.e. it encodes the same information content as the flattened space in figure 1 B, although again this is a topological rather than a topographical isomorphism. The warping of this space would not be apparent to the percipient, because the very definition of straightness is warped along with the space itself, as suggested by the warped grid lines in the figure. In contrast, consider the flattened representation depicted in figure 1 D, where the perceptual representation has been segmented into discrete depth planes, that distinguish only foreground from background objects. This model is no longer isomorphic with the perceptual experience it supposedly represents, because unlike this model, the perceptual experience manifests a specific and distinct depth value for every point in each of the surfaces of the percept. Furthermore the perceptual experience manifests an experience of empty space surrounding the perceived objects, every point of which is experienced simultaneously and in parallel as a volumetric continuum of a certain spatial resolution, whereas the model depicted in figure 1 D encodes only a small number of discrete depth planes. This kind of model therefore is inadequate as a perceptual model of the information content of conscious experience, because the dimensions of its representation are less than the dimensions of the experience it attempts to model.
Now a functional isomorphism must also preserve the functional transformations observed in perception, and the exact requirements for a functional isomorphism depend on the functionality in question. For example when a colored surface is perceived to translate coherently across perceived space, the corresponding color values in the perceptual representation of that surface must also translate coherently through the perceptual map. If that memory is discontinuous, like a digital image distributed across separate memory chips on a printed circuit board, then the perceptual representation of that moving surface must jump seamlessly across those discontinuities in order to account for the subjective experience of a continuous translation across the visual field. In other words a functional isomorphism requires a functional connectivity in the representation as if a structurally isomorphic memory were warped, distorted, or fragmented while preserving the functional connectivity between its component parts. Consider for example a representational mechanism as shown in figure 1 A, equipped with additional computational hardware capable of performing spatial transformations on the volumetric image in the representation. For example the representational mechanism might be equipped with functions which can rotate, translate, and scale the spatial pattern in the representation on demand. This representation would thereby be invariant to rotation, translation, and scale, because the spatial pattern of the block itself is encoded independent of its rotation, translation, and scale. The fact that an object in perception maintains its structural integrity and recognized identity despite rotation, translation, and scaling by perspective, is clear evidence for this kind of invariance in human perception and recognition. If the warped model shown in figure 1 C were also equipped with these same transformational functions, the warped representation would also be functionally isomorphic with the non-warped representation, as long as those transformations are performed correctly with respect to the warped geometry of that space. A functional isomorphism is even possible for a representation which is fragmented into separate pieces, so long as those pieces are wired together in such a way as to continue to perform the spatial transformations exactly the same as in the corresponding undistorted mechanism. A functional isomorphism can even survive in a volumetric representation whose individual elements or voxels are scrambled randomly across space, so long as the functional connections between those elements are preserved through the scrambling. The result is a representation which is neither topographically nor topologically isomorphic with the perceptual experience it represents. However it remains a volumetric representation, with an explicit encoding of each point in the represented space to a particular spatial resolution, and it remains functionally isomorphic with the spatial experience that it represents, capable of performing coherent rotation, translation, and scaling transformations of the perceptual structures expressed in the representation.
An explicit volumetric spatial representation capable of spatial transformation functions as described above, is more efficiently implemented in either a topographically isomorphic, or a topologically isomorphic form, which require shorter and more orderly connections between adjacent elements in the representation. However the argument for structural or topological isomorphism is an argument of representational efficiency and simplicity, rather than of logical necessity. A functional isomorphism on the other hand is strictly required in order to account for the properties of the perceptual world as observed subjectively. The volumetric structure of visual consciousness, and perceptual invariance to rotation, translation, and scale, offer direct and concrete evidence for an explicit volumetric spatial representation in the brain, which is at least functionally isomorphic with the corresponding spatial experience.
A neurophysiological model of perceptual processing and representation should concern itself with the actual mechanism in the brain. In the case of a distorted representation as suggested in figure 1 C the warping of that perceptual map would be a significant feature of the model. A perceptual model on the other hand is concerned with the structure of the percept itself, independent of any warping of the representational manifold. Even for a representation which is functionally but not structurally isomorphic, a description of the functional transformations performed in that representation are most simply expressed in their structurally isomorphic form, just as a panning or scrolling function in image data is most simply expressed as a spatial shifting of image data, even when that shifting is actually performed in hardware in a non-isomorphic memory array. Therefore the functional operation of a warped mechanism like figure 1 C is most simply described as the operation of the functionally equivalent undistorted mechanism in figure 1 A. In the present discussion therefore, our concern will be chiefly with the functional architecture of perception, i.e. a description of the spatial transformations observed in perception, whatever form those transformations might take in the physical brain, and those transformations are most simply described as if taking place in a physically isomorphic space.
In the discussion that follows, the terminology "spatial representation", "data expressed in spatial form", "literal volumetric replica of the world inside your head", "three-dimensional pattern of opaque state units", "explicit three-dimensional replica of the surface", and "volumetric spatial medium," will refer not to a topographically isomorphic model of space, as suggested in figure 1 A, but to a functionally isomorphic model of space like the warped model in figure 1 C, in which the explicit volumetric representation is possibly warped and distorted, but still encodes an explicit value for every volumetric point in perceived space as well as the neighborhood relations between those values. This is in contrast to the more commonly assumed flattened or abstracted cortical representation depicted in figure 1 D, where the volumetric mapping is no longer preserved.
The issue of isomorphism is so profoundly problematic for theories of perceptual representation that theorists have gone to no end of trouble in an effort to dispel the issue, and to argue that isomorphism is not actually necessary. A careful examination of these proposals however reveals the naïve realist assumptions on which they are founded.
Shepard and Chipman (1970) argue that when we perceive a square, for example, that there is no need for an internal perceptual replica of that square in the brain of the percipient. They argue that we learn the appropriate use of words such as "square" from a verbal community that has access only to the public object and not to any such private image. If there is some internal event that corresponds to our experience of a square, whether it be the activation of a cell or cell assembly in the brain, our ability to form an association between this event and the word "square" requires only that this event have a regular relation to the external object of causality, not of structural isomorphism. To insist, in addition, that these neurons must be spatially arranged in precisely the form of a square, does not in the least help to explain how they come to trigger the naming response "square," at least according to Shepard and Chipman.
In this brief introductory paragraph Shepard and Chipman neatly turn the tables on the debate by characterizing the perception of a square as the issue of learning the naming response "square", which is an issue of recognition, rather than perception. To be sure, recognition is an important aspect of perception, and the problem of learning a naming response is a formidable one that deserves further investigation. But the recognition response is by no means the same thing as the perceptual experience of the square as a continuous filled-in square-shaped region of sense-data experienced in the visual field. How can so intelligent and educated researchers come to make such a profound error in identification of the issue at hand? The answer is clear from their assertion that a verbal community has access only to the public object, and not to any private image. This naïve realist assumption is passed off casually as a statement of fact, but in fact it reveals an implicit commitment to the notion that the three-dimensional volumetric objects that we observe to occupy the space of our perceptual field, are the actual objects themselves, and that therefore they need not be replicated or re-represented again in the brain. The fact that this assumption goes unchallenged, and even largely unnoticed by the community at large, demonstrates how deeply the assumptions of naïve realism have become entrenched in contemporary thought.
Shepard (1981) makes another attempt to dispel the issue of isomorphism by arguing for psychophysical complementarity rather than isomorphism. Appropriately enough, Shepard cites that grand master of naïve realism, B. F. Skinner, who argued that even if we were to discover a part of the brain in which the physical pattern of neural activity had the very same shape as the corresponding external object—say a square—we would not in this way have made any progress in explaining how the subject is able to recognize that object as a square, or to learn to associate to it a unique verbal response "square." So again the issue of perception is confounded with the issue of recognition response. Skinner's statement is true enough, as far as it goes. But what Shepard and Skinner fail to acknowledge is that it would be very much harder to learn to recognize a square if you could not "see" it, i.e. if you did not have direct access to an internal representation of the square as a square shaped sense-datum to associate with the appropriate recognition response. To claim that we can experience the square without such an internal replica is just plain magic. Furthermore, until we do discover a part of the brain in which the physical pattern of neural activity (or some other physically measurable quantity) had the very same shape as the corresponding external object, the phenomenal aspect of that volumetric spatial structure remains as a nomological dangler, something that is experienced as a spatial picture, something that is clearly distinct from the actual square in the real world (especially when that square is illusory), but that does not actually exist in any space known to science. Like the Behaviorists before him, Shepard attempts to discount the entire ediface of conscious experience as if it simply did not exist as a scientific entity.
There is a further difficulty with this notion of psychophysical complementarity. Shepard argues that the relation of the mental representation to the external object it represents might be one of complementarity, rather than one of similarity or resemblance. Just as a lock has a hidden structure that is to some extent complementary to the visible contour of the key that fits it, Shepard argues the internal structure that is uniquely activated by a given object must have a structure that somehow meshes with the pattern manifested by its object, i.e. the "shape" of the representation is complementary to, rather than isomorphic with the object that it represents. But again, this notion of perceptual representation is only coherent from a naïve realist perspective. For if we interpret this argument from an indirect perceptual view, it would have to be the square shape that we experience in immediate consciousness that is complementary to the external square, which is beyond our direct experience. In other words, the real "square" in the external world is not actually square, as we observe it to be, but rather it would have to be somehow complementary to the square shape that we observe in conscious experience, an idea that is obviously absurd.
In yet another, somewhat different defense of naïve realism, Shepard argues (1981 p. 292) that the relation between the external object and its internal representation might be a kind of paramorphism rather than isomorphism, as seen for example in the Fourier transform of an image, which encodes all of the information in a spatial image but in a very abstract non-spatial form. Again, this argument is founded on the naïve assumption that the world we see around us is the world itself, and that therefore the paramorphic representation of that world is not identified as the image of the world we see around us, but as our verbal or conceptual recognition of that world. For if the perceptual brain did employ a Fourier representation instead of a spatial one, then the world we see around us would necessarily appear in the form of a Fourier transform rather than as a spatial structure, which again, is obviously absurd. The fact that the world around us appears as a volumetric spatial structure is direct and concrete evidence for a spatial representation in the brain. What is most interesting about this issue is that Shepard clearly does not fully comprehend the position that he challenges, and therefore his criticisms of isomorphism inevitably miss the mark.
Steven Palmer (1999) on the other hand strikes at the very heart of the issue of isomorphism. Palmer draws a distinction between two different aspects of conscious experience, the intrinsic qualities of experiences themselves versus the relational structure that holds among those experiences. The intrinsic qualities, such as the color qualia in the experience of color, are in principle impossible to communicate from one mind to another, and therefore they are inaccessible to science (except through phenomenology), a restriction that Palmer calls the subjectivity barrier. All that can be communicated about conscious experience is the relational structure that holds among those experiences. In the case of color experience for example, subjects say that orange is more similar to red than it is to green or blue, and that aqua is experienced as intermediate between green and blue, etc. It was exactly these relational facts of color experience that were used to define the "color solid" in the CIE chromaticity diagram. A relational structure like the color solid encodes a great deal of information implicitly about the relations between its variables in a manner that is practically impossible to express as explicit relations, because the number of binary, trinary, and other relations between colors implicitly expressed in the color solid is so astronomical as to defy any kind of exhaustive listing or discrete associative links. And yet all of those relations are evidently available to the psychophysical subject when making phenomenal color judgements. This strongly suggests that the variables of phenomenal color experience are encoded in the brain as a relational structure whose information content is identical to that of the color solid, rather than as a list of the astronomical number of relations between individual colors that are expressed implicitly within the color solid.
The subjectivity barrier is often cited as an insurmountable obstacle to meaningful phenomenological examination of brain states. But Palmer observes that the isomorphism constraint goes both ways. Not only is it impossible to express intrinsic color experience in objective external terms, but even if there were some way to quantify the intrinsic qualities of experience, it would then be impossible to infer the structure of the brain from that intrinsic information. The relational structure on the other hand does offer direct evidence for the dimensions of color experience as expressed in the physical brain, because relational information is the only information that can cross the subjectivity barrier in either direction. Palmer's analysis of isomorphism has profound implications not only for color perception, but also for the perception of space, although curiously Palmer avoids discussing the issue of spatial perception, presumably so as not to emperil his chances of getting his paper published with such a controversial thesis. But a spatial percept, like that of a square, is clearly a relational structure, in the sense that every point of the percept is presented simultaneously in proper spatial relation to every other point in the square. In other words our experience of a square is of a spatial structure, and therefore the information encoded in spatial perception is an explicit spatial one, whether expressed in topographical or only topological isomorphic form.
The phenomenal world is composed of solid volumes, bounded by colored surfaces, embedded in a spatial void. Every point on every visible surface is perceived at an explicit spatial location in three-dimensions (Clark 1993, Lehar 2003), and all of the visible points on a perceived object like a cube or a sphere, or this page, are perceived simultaneously in the form of continuous surfaces in depth. The perception of multiple transparent surfaces, as well as the experience of empty space between the observer and a visible surface, reveals that multiple depth values can be perceived at any spatial location. I propose to model the information in perception as a computational transformation from a two-dimensional colored image, (or two images in the binocular case) to a three-dimensional volumetric data structure in which every point can encode either the experience of transparency, or the experience of a perceived color at that location. The appearence of a color value at some point in this representational manifold corresponds by definition to the subjective experience of that color at the corresponding point in phenomenal space. If we can describe the generation of this volumetric data structure from the two-dimensional retinal image as a computational transformation, we will have quantified the information processing apparent in perception, as a necessary prerequisite to the search for a neurophysiological mechanism that can perform that same transformation.
This "picture-in-the-head" or "Cartesian theatre" concept of visual representation has been criticized on the grounds that there would have to be a miniature observer to view this miniature internal scene, resulting in an infinite regress of observers within observers (Dennett 1991, 1992, O'Regan 1992, Pessoa et al. 1998). In fact there is no need for an internal observer of the scene, since the internal representation is simply a data structure like any other data in a computer, except that this data is expressed in spatial form (Earle 1998, Singh & Hoffman 1998, Lehar 2003). For if a picture in the head required a homunculus to view it, then the same argument would hold for any other form of information in the brain, which would also require a homunculus to read or interpret that information. In fact any information encoded in the brain needs only to be available to other internal processes rather than to a miniature copy of the whole brain. The fact that the brain does go to the trouble of constructing a full spatial analog of the external environment merely suggests that it has ways to make use of this spatial data. For example field theories of navigation have been proposed (Koffka 1935 pp 42-46, Gibson & Crooks, 1938) in which perceived objects in the perceived environment exert spatial field-like forces of attraction and repulsion, drawing the body towards attractive percepts, and repelling it from aversive percepts, as a spatial computation taking place in a spatial medium. If the idea of an explicit spatial representation in the brain seems to "fly in the face of what we know about the neural substrates of space perception" (Pessoa et al. 1998 author's response R3.2 p. 789), it is our theories of spatial representation that are in urgent need of revision. For to deny the spatial nature of the perceptual representation in the brain is to deny the spatial nature so clearly evident in the world we perceive around us. To paraphrase Descartes, it is not only the existence of myself that is verified by the fact that I think, but when I experience the vivid spatial presence of objects in the phenomenal world, those objects are certain to exist, at least in the form of a subjective experience, with properties as I experience them to have, i.e. location, spatial extension, color, and shape. I think them, therefore they exist (Price 1932, p. 3). All that remains uncertain is whether those percepts exist also as objective external objects as well as internal perceptual ones, and whether their perceived properties correspond to objective properties. But their existence and fully spatial nature in my internal perceptual world is beyond question if I experience them so, even if only as a hallucination.
The idea of perception as a literal volumetric replica of the world inside your head immediately raises the question of boundedness, i.e. how an explicit spatial representation can encode the infinity of external space in a finite volumetric system. The solution to this problem can be found by inspection. For phenomenological examination reveals that perceived space is not infinite, but is bounded (Lehar 2003). This can be seen most clearly in the night sky, where the distant stars produce a dome-like percept that presents the stars at equal distance from the observer, and that distance is perceived to be less than infinite. The lower half of perceptual space is usually filled with a percept of the ground underfoot, but it too becomes hemispherical when viewed from far enough above the surface, for example from an airplane or a hot air balloon. The dome of the sky above, and the bowl of the earth below therefore define a finite approximately spherical space (Heelan 1983) that encodes distances out to infinity within a representational structure that is both finite and bounded. While the properties of perceived space are approximately Euclidean near the body, there are peculiar global distortions evident in perceived space that provide clear evidence of the phenomenal world being an internal rather than external entity.
Consider the phenomenon of perspective, as seen for example when standing on a long straight road that stretches to the horizon in a straight line in opposite directions. The sides of the road appear to converge to a point both up ahead and back behind, but while converging, they are also perceived to pass to either side of the percipient, and at the same time, the road is perceived to be straight and parallel throughout its entire length. This property of perceived space is so familiar in everyday experience as to seem totally unremarkable. And yet this most prominent violation of Euclidean geometry offers clear evidence for the non-Euclidean nature of perceived space. For the two sides of the road must therefore in some sense be perceived as being bowed, and yet while bowed, they are also perceived as being straight. This can only mean that the space within which we perceive the road to be embedded, must itself be curved. In fact, the observed warping of perceived space is exactly the property that allows the finite representational space to encode an infinite external space. This property is achieved by using a variable representational scale, i.e. the ratio of the physical distance in the perceptual representation relative to the distance in external space that it represents. This scale is observed to vary as a function of distance from the center of our perceived world, such that objects close to the body are encoded at a larger representational scale than objects in the distance, and beyond a certain limiting distance the representational scale, at least in the depth dimension, falls to zero, i.e. objects beyond a certain distance lose all perceptual depth. This is seen for example where the sun and moon and distant mountains appear as if cut out of paper and pasted against the dome of the sky.

The distortion of perceived space is suggested in figure 2 which depicts the perceptual representation for a man walking down a road. The phenomenon of perspective is by definition a transformation defined from a three-dimensional world through a focal point to a two-dimensional surface. The appearence of perspective on the retinal surface therefore is no mystery, and is similar in principle to the image formed by the lens in a camera. What is remarkable in perception is the perspective that is observed not on a two-dimensional surface, but somehow embedded in the three-dimensional space of our perceptual world. Nowhere in the objective world of external reality is there anything that is remotely similar to the phenomenon of perspective as we experience it phenomenologically, where a perspective foreshortening is observed not on a two-dimensional image, but in three dimensions on a solid volumetric object. The appearence of perspective in the three-dimensional world we perceive around us is perhaps the strongest evidence for the internal nature of the world of experience, for it shows that the world that appears to be the source of the light that enters our eye, must actually be downstream of the retina, for it exhibits the traces of perspective distortion imposed by the lens of the eye, although in a completely different form.
This view of perspective offers an explanation for another otherwise paradoxical but familiar property of perceived space whereby more distant objects are perceived to be both smaller, and yet at the same time to be perceived as undiminished in size. This corresponds to the difference in subject's reports depending on whether they are given objective v.s. projective instruction (Coren et al., 1994. p. 500) in how to report their observations, showing that both types of information are available perceptually. This duality in size perception is often described as a cognitive compensation for the foreshortening of perspective, as if the perceptual representation of more distant objects is indeed smaller, but is somehow labeled with the correct size as some kind of symbolic tag representing objective size attached to each object in perception. However this kind of explanation is misleading, for the objective measure of size is not a discrete quantity attached to individual objects, but is more of a continuum, or gradient of difference between objective and projective size, that varies monotonically as a function of distance from the percipient. In other words, this phenomenon is best described as a warping of the space itself within which the objects are represented, so that objects that are warped coherently along with the space in which they are embedded appear undistorted perceptually. The mathematical form of this warping will be discussed in more detail below.
This model of spatial representation emphasizes another aspect of perception that is often ignored in models of vision, that our percept of the world includes a percept of our own body within that world, and our body is located at a very special location at the center of that world, and it remains at the center of perceived space even as we move about in the external world. Perception is embodied by its very nature, for the percept of our body is the only thing that gives an objective measure of scale in the world, and a view of the world around us is useless if it is not explicitly related to our body in that world. The little man at the center of the spherical world of perception therefore is not a miniature observer of the internal scene, but is itself a spatial percept, constructed of the same perceptual material as the rest of the spatial scene, for that scene would be incomplete without a replica of the percipient's own body in his perceived world. Gibson was right therefore in his emphasis on the interaction of the active organism with its environment. Gibson's only error was the epistemological one, for Gibson failed to recognize that the organism and its environment that are active in perception are themselves internal perceptual replicas of their external counterparts. It was this epistemological confusion that led to the bizarre aspects of Gibson's otherwise valuable theoretical contributions.
Indirect realism offers direct evidence for a spatial representation in the brain. But there remains one final question regarding the ultimate nature of consciousness. Even if there is a spatial representation in the brain, why should it be conscious of itself? Why should it not behave much like a machine, that performs its function using either a spatial or a symbolic principle of computation, but presumably the machine performs its function without any conscious experience of what it is doing. Why should human consciousness be any different?
But there is a large unstated assumption implied in the very framing of this question. That is the assumption that a machine could not possibly be conscious. This assumption is generally taken for granted, because the alternative, that everything in the universe must have some primitive level of consciousness, seems so absurd from the outset that, like solipsism, we tend to discount it even if we cannot disprove it on logical grounds. But can we really be sure that this alternative is so absurd? Obviously, like solipsism, the possibility of panpsychism, or more likely, panexperientialism (Chalmers 1995, Rosenberg 2002) is a question that might never be provable one way or the other. Nevertheless, it is of vital importance that we get this question right, because if we come down on the wrong side of this paradigmatic fence, that will necessarily throw all the rest of our philosophy completely out of kilter.
If we accept the materialist view that mind is a physical process taking place in the physical mechanism of the brain, and since we know that mind is conscious, then that already is direct and incontrovertible evidence that a physical process taking place in a physical mechanism can under certain conditions be conscious. Now it it true that the brain is a very special kind of mechanism. But what makes the brain so special is not its substance, for it is made of the ordinary substance of matter and energy. What sets the brain apart from normal matter is its complex organization. The most likely explanation therefore is that what makes our consciousness special is not its substance, but its complex organization. The fundamental "stuff" of which our consciousness is composed, i.e. the basic qualia of color and pain, sadness and joy, are apparently common with the qualia of children, as far back as I can remember, although I also remember a less complex organization of my experiences as a child. It is also likely that animals have some kind of conscious qualia on logical grounds, because the information of their perceptual experience cannot exist without some kind of carrier to express that information in the physical brain. Whether the subjective qualia of different species, or even different individuals of our own species, are necessarily the same as ours experientially, is a question that is difficult or maybe impossible in principle to answer definitively. But the simplest, most parsimonious explanation is that our own conscious qualia evolved from those of our animal ancestors, and differ from those earlier forms more in its level of complex organization rather than in its fundamental nature.
The natural reluctance that we all feel to extending consciousness to our animal ancestors, and even more so to plants, or to inanimate matter, is a stubborn legacy of our anthropocentric past. But the history of scientific discovery has been characterized by a regular progression of anthrodecentralization, demoting humans from the central position in the universe under the personal supervision of God, to lost creatures on the surface of a tiny blip of matter orbiting a very unremarkable star, among countless billions of stars in an unremarkable galaxy amongst countless billions of other galaxies as far as the telescopic eye can see. Modern biology has now discovered that there is no vital force in living things, but only a complex organization of the ordinary matter of the universe, following the ordinary laws of that universe. There is no reason on earth why consciousness should not also be considered to be a manifestation of the ordinary matter of the universe following the ordinary laws of that universe, although expressed in a complex organization in the case of the human brain. A claim to the contrary would necessarily fall under the category of an extraordinary claim, which, as Carl Sagan pointed out, would require extraordinary evidence for it to be accepted by reasonable men.
When we examine the chain of biocomplexity from the simplest pure chemical to the most complex human brain, there is a continuous progression from single atoms, to simple compound molecules, to complex organic molecules, to proteins and DNA, to viruses, to simple single-celled organisms, and all the way up the evolutionary chain to the brain of man. If we are to claim that consciousness is uniquely human, or unique to animals of a certain complexity, then there would necessarily be some kind of abrupt transition along that progression where that consciousness comes suddenly into existence, and that abrupt transition would occur both for the individual during gestation, and for the species, during evolution. The claim that consciousness is unique to humans, or to animals, or to living creatures, is bedeviled by the fact that there are always transitionary forms to be found, that are intermediate between humans and animals, between animals and plants, and between living and non-living creatures such as hypercomplex molecules like viruses, and there is also a continuous progression during gestation from fertilized egg to full human body. If we posit that consciousness appears abruptly at any one of these transitions where the only observed difference is a slight increase in complexity of organization, is again to lapse into nomological danglers and vital force, because the postulated conscious quality that supposedly appears abruptly at that point is undetectable to science, and therefore it is a quality of the supervenient spirit world rather than anything knowable by, or demonstrable to science. Surely the time has come to finally accept the full implications of Darwin's theory of evolution, and acknowledge the fact that our nature and our consciousness are not of a separate spiritual realm, but are composed of the very same material substance and energy of which the rest of the universe is composed.
The inescapable conclusion is that all matter and energy have some kind of primal proto-consciousness, what Chalmers (1995) calls panexperientialism to distinguish it from panpsychism, the view that everything is conscious in any human kind of sense. The more plausible panexperientialism posits merely that there exists a very simple proto-consciousness in inanimate matter that is a fundamental property of that matter. For inanimate matter, this proto-consciousness is something so simple and primitive that we would hardly recognize it as consciousness at all. And yet when this proto-consciousness is organized in the right manner in a human brain, it gives rise to the wonderful splendour of human consciousness. We are not external observers of the physical universe, we ourselves are part of that universe, and our experience is a tiny fragment of the experience of the larger universe around us, although expressed in a very much more complex form in the human brain. This way of describing consciousness is the only true monism, that really equates mind with the functioning of physical matter, without recourse to nomological danglers and spiritual mumbo-jumbo.
This identity relation between mind and matter casts a new light on Searle's (1997) assertion that "a computer is not even a computer to a computer." What would the consciousness of a computer be like, if a computer did have consciousness? Consider the hypothesis that consciousness is a manifestation of forces and energy, or energetic wrinkles in space-time, or what Rosenberg (2002) calls manifestations of causality in the physical world. The consciousness of a computer would thereby correspond to the patterns of energy in its chips and wires. In a digital computer that consciousness would be a very binary affair, and it is also in the very nature of digital computation that complex calculations are divided into a number of very simple steps, each of which can be computed independent of the problem as a whole. The consciousness of a computer would thereby be a very fragmented kind of thing, with each flip-flop or logic gate experiencing only the energy state in its local inputs and outputs, since those are the only forces that influence the local logic gate. There is a very different kind of energy structure in an analog spatial system like a soap bubble, whose entire surface is under tension against the outward pressure of the captured air. A push on any point of the bubble has an immediate influence on the bubble as a whole, on the entire gestalt, whose causal structure works in an emergent manner to try to restore the spherical shape. If a soap bubble has any form of primal consciousness, that proto-consciousness would be of an elastic spherical form under stress, as a unitary gestalt.
It is curious that Searle, in his Chinese room analogy, (Searle 1980) assumes a fragmented rule-based mechanism as his model of conscious experience, because in his analogy the Chinese translation is performed step by step very much like the computation in a digital computer. No wonder there is no emergent global consciousness from such a fragmented computational analogy. Consider by contrast the phenomenon known as "the wave" that occasionally erupts spontaneously in a crowd of excited spectators at a sports event. The "algorithm" for each individual is very simple and local—observe and mimic the orientation of the raised arms in the crowd you see around you. As if by magic, what appears as just a local swaying of arms, when observed from a distance appears as larger waves of synchronized motion sweeping through the crowd. It is as if some larger global animated entity had suddenly come into existence, of which the local elements are only vaguely aware in a piecewise manner, and yet every aspect of that animated wavelike structure is exactly determined by its local elementary components. But does the larger wavelike entity have a corresponding global consciousness independent of the individual consciousnesses of its constituent parts? And does that larger consciousness include the consciounsesses of its individual parts? The answer to those questions can be found by inspection of our own consciousness. By the fact that we ourselves have global consciousness, we can infer that larger global phenomena in the brain do give rise to global emergent consciousness that takes the form we observe in the perceived world around us. And that global consciousness does not appear to include a consciousness of its individual elements, for we are completely unaware of the component electrons, molecules, and neurons of our own physical brain that must be responsible for that global percept. Our personal conscious experience is therefore confined to an awareness of the spatial structures of the patterns of energy in our brain, although presumably there would also be many more independent and disconnected consciousnesses in the energy structures of our physical body of which we are not directly aware, and most likely there are also multiple independent conscious entities within our own brain, that make up the "unconscious mind," of which our central narrative consciousness is not directly aware.
Consider the experience of swallowing food. I am conscious of the inside of my mouth as a vivid three-dimensional structure "colored" by sensations of taste and texture, warmth and cold. But this spatial consciousness terminates abruptly at the threshold of my throat, beyond which my spatial consciousness of the food is abruptly cut off. But the rest of my alimentary canal performs wave-like motions of peristaltic contraction, very much like the kind of manipulation that occurs consciously in my mouth, all beyond my own personal conscious awareness. Is my alimentary canal conscious of itself, or does it perform its function totally in the absence of conscious experience? If the food that I swallowed was a hot and spicy Vindaloo curry, I know in an indirect way that my stomach is feeling the burning pain because I can feel it churning and grinding in protest, although I cannot feel its pain directly, only remotely, like a loud argument heard through the wall in an adjacent motel room. And the next morning, as the Vindaloo curry passes another abrupt threshold portal, I become suddenly aware of the pain again as part of my own personal experience. It seems that conscious experience has a direct functional role, because my consciousness of my own mouth helps me to chew the food and direct it intelligently down my throat without choking. Without conscious experience I could no longer perform this remarkable feat, and could only be fed with a feeding tube. The simplest explanation therefore is that my alimentary canal has a similar conscious experience, i.e. it feels the waves of peristaltic contraction which are its own conscious wave-like thoughts, just as I feel the inside of my mouth, although unlike my central narrative consciousness, presumably the alimentary consciousness is not burdened by memories or aspirations, or any real self-consciousness except of itself as a spatial structure, and of the vital imperative to propel arriving food further down the pipeline. This hypothesis is supported by the fact that there exist rare individuals who have conscious control over their own bowel function, such that they can consciously control their own alimentary peristaltic contractions just as we can control the contractions of our mouth. To claim that conscious experience is an epiphenomenon with no functional value is to relegate that experience to a nomological dangler forever beyond scientific scrutiny.
There is not, therefore, a single "bridge locus" as the only place in the brain where consciousness occurs, but rather there is one global representational mechanism that has verbal and cognitive access to the components of ordinary consciousness including memories and aspirations, and then there are countless additional independent conscious energy structures disconnected from our global or narrative consciousness of which we remain personally unaware. Each of those islands of consciousness has an isolated experience of its own energy structure.
If consciousness is indeed identical to energy structure, then the spherical bubble can be conscious of its own spherical form, although it has neither memory nor aspirations, nor any kind of understanding, except an understanding of its own spherical energy structure. But how then does human consciousness come to be aware not only of its own structure, but also that of the external environment around it beyond the bounds of the physical brain? It does so by constructing a much more complex and elaborate bubble structure in the human brain, composed of patterns of electrochemical energy that take the form of a replica of the external world, complete with a replica of our own body at the center of that representational space. So in answer to Searle's contention, the computer too could acquire a consciousness of itself, if it were loaded with a representation of itself. The pattern of that representation in the computer would thereby appear as a computer to the computer. Of course like we ourselves, the computer would not notice that what it was seeing was not really an image of it's real self, as viewed from the outside, but merely a miniature representation of itself that is entirely contained within itself, because its computational consciousness cannot extend beyond the confines of its computational brain. The computer would not know that everything of which it is aware is actually surrounded by the larger physical computer, which in turn is composed of entirely different and independent sets of conscious energy structures in the physical structures of its frame and screws and power supply.
If this notion of panexperientialism, or proto-consciousness of inanimate matter, sounds bizarre and far-fetched, we should bear in mind that whatever the ultimate solution to the mind-brain quandary, it is sure to do considerable violence to our normal everyday common sense notions of reality. When it comes to these fundamental issues of existence, our intuitive instincts are almost certain to fail us, and therefore every alternative should be given serious consideration however implausible it might at first seem intuitively. For as intuitively incredible as the notion of panexperientialism might seem, the alternatives are all fraught with even more profound philosophical paradoxes and contradictions. But whatever our theoretical inclinations on the ultimate question of consciousness, it is important to point out that this is a separate and independent issue from the question of whether the internal representation of the brain is spatial or symbolic. Whichever way the answer to the ultimate question goes, whether consciousness is uniquely human, or is shared with the living and non-living worlds, unless we wish to believe in some magical nomological dangler that extends mind half way into the spirit world, we must face the observational fact that there is a spatial representation in the brain.
One of the most formidable obstacles facing computational models of the perceptual process is that perception exhibits certain global Gestalt properties such as emergence, reification, multistability, and invariance that are difficult to account for either neurophysiologically, or even in computational terms such as computer algorithms. The ubiquity of these properties in all aspects of perception, as well as their preattentive nature suggests that Gestalt phenomena are fundamental to the nature of the perceptual mechanism. I propose that no useful progress can possibly be made in our understanding of neural processing until the computational principles behind Gestalt theory have been identified.
Figure 3 shows a picture that is familiar in vision circles, for it reveals the principle of emergence in a most compelling form. For those who have never seen this picture before, it appears initially as a random pattern of irregular shapes. A remarkable transformation is observed in this percept as soon as one recognizes the subject of the picture as a dalmation dog in patchy sunlight in the shade of overhanging trees. What is remarkable about this percept is that the dog is perceived so vividly despite the fact that much of its perimeter is missing. Furthermore, visual edges which form a part of the perimeter of the dog are locally indistinguishable from other less significant edges. Therefore any local portion of this image does not contain the information necessary to distinguish significant from insignificant edges.

Although Gestalt theory did not offer any specific computational mechanism to explain emergence in visual perception, Koffka (1935) suggested a physical analogy of the soap bubble to demonstrate the operational principle behind emergence. The spherical shape of a soap bubble is not encoded in the form of a spherical template or abstract mathematical code, but rather that form emerges from the parallel action of innumerable local forces of surface tension acting in unison. The characteristic feature of emergence is that the final global form is not computed in a single pass, but continuously, like a relaxation to equilibrium in a dynamic system model. In other words the forces acting on the system induce a change in the system configuration, and that change in turn modifies the forces acting on the system. The system configuration and the forces that drive it therefore are changing continuously in time until equilibrium is attained, at which point the system remains in a state of dynamic equilibrium, i.e. its static state belies a dynamic balance of forces ready to spring back into motion as soon as the balance is upset.
Emergence is actually the issue that inspired Davidson's (1970) theory of anomalous monism. Davidson argues (p. 247) that mental events resist capture in the nomological net of physical theory. For mentalistic propositions do not display the lawlike character of physical ones: Davidson asserts, (p. 248) "there are no strict deterministic laws on the basis of which mental events can be predicted and explained," and this is the principle of the anomalism of the mental. But Wolfgang Köhler (1924) showed that in fact there is no magic in emergence, emergence is a common property of certain kinds of physical systems, such as the soap bubble taking on its spherical shape, or water seeking its own level in a vessel, or of global weather patterns which cannot be lawfully predicted from their present state. To insist that mind supervenes on the brain in some mysterious way, is like saying that the soap bubble supervenes on soapy water, or that the water level supervenes on the body of water in a vessel, or that global weather patterns supervene on the earth's physical atmosphere. But this is no different than saying that these are emergent processes that are already the simplest model of themselves. Emergence in perception does not imply that the mind supervenes on the brain, but rather it indicates that the neurophysiological processes involved in perception exhibit the kind of holistic emergence seen in the soap bubble, where a multitude of tiny forces act together simultaneously to produce a final perceptual state by way of a process which cannot be reduced to simple laws.
The Kanizsa figure (Kanzsa 1979) shown in figure 4 A, is one of the most familiar illusions introduced by Gestalt theory. In this figure the triangular configuration is not only recognized as being present in the image, but that triangle is filled-in perceptually, producing visual edges in places where no edges are present in the input, and those edges in turn are observed to bound a uniform triangular region that is brighter than the white background of the figure. Idesawa (1991) and Tse (1999a, 1999b) have extended this concept with a set of even more sophisticated illusions such as those shown in Figure 4 B through D, in which the illusory percept takes the form of a three-dimensional volume. These figures demonstrate that the visual system performs a perceptual reification, i.e. a filling-in of a more complete and explicit perceptual entity based on a less complete visual input. Reification is a general principle of perceptual processing, of which boundary completion and surface filling-in are more specific computational components. The identification of this generative aspect of perception was one of the most significant contributions of Gestalt theory.
A familiar example of multistability in perception is seen in the Necker cube, shown in Figure 5 A. Prolonged viewing of this stimulus results in spontaneous reversals, in which the entire percept is observed to invert in depth. Figure 5 B shows how large regions of the percept invert coherently in bistable fashion. Even more compelling examples of multistability are seen in surrealistic paintings by Salvator Dali, and etchings by Escher, in which large and complex regions of the image are seen to invert perceptually, losing all resemblance to their former appearence (Attneave 1971). The significance for theories of visual processing is that perception cannot be considered as simply a feed-forward processing performed on the visual input to produce a perceptual output, as it is most often characterized in computational models of vision, but rather perception must involve some kind of dynamic process whose stable states represent the final percept.
A central focus of Gestalt theory was the issue of invariance, i.e. how an object, like a square or a triangle, can be recognized regardless of its rotation, translation, or scale, or whatever its contrast polarity against the background, or whether it is depicted solid or in outline form, or whether it is defined in terms of texture, motion, or binocular disparity. This invariance is not restricted to the two-dimensional plane, but is also observed through rotation in depth, and even in invariance to perspective transformation. For example the rectangular shape of a table top is recognized even when its retinal projection is in the form of a trapezoid due to perspective, and yet when viewed from any particular perspective we can still identify the exact contours in the visual field that correspond to the boundaries of the perceived table, to the highest resolution of the visual system. The ease with which these invariances are handled in biological vision suggests that invariance is fundamental to the visual representation.
Our failure to find a neurophysiological explanation for Gestalt phenomena does not suggest that no such explanation exists, only that we must be looking for it in the wrong places. The enigmatic nature of Gestalt phenomena only highlights the importance of the search for a computational mechanism that exhibits these same properties. In the next section I present a model that demonstrates how these Gestalt principles can be expressed in a computational model that is isomorphic with the subjective experience of vision.
The basic function of visual perception can be described as the transformation from a two-dimensional retinal image, or a pair of images in the binocular case, to a solid three-dimensional percept. Figure 6 A depicts a two-dimensional stimulus that produces a three-dimensional percept of a solid cube complete in three dimensions. For simplicity, a simple line drawing is depicted in the figure, but the argument applies more appropriately to a view of a real cube observed in the world. Every point on every visible surface of the percept is experienced at a specific location in depth, and each of those surfaces is experienced as a planar continuum, with a specific three-dimensional slope in depth. The information in this perceptual experience can therefore be expressed as a three-dimensional model, as suggested in figure 6 B, constructed on the basis of the input image in figure 6 A.
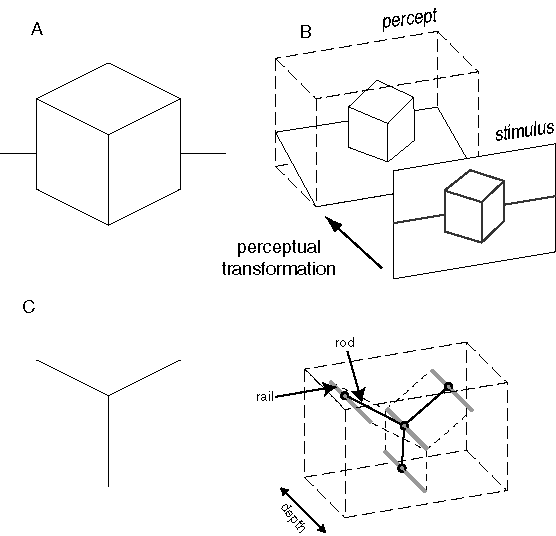
The transformation from a two-dimensional image space to a three-dimensional perceptual space is known as the inverse optics problem, since the intent is to reverse the optical projection in the eye, in which three-dimensional information from the world is collapsed into a two-dimensional image. However the inverse optics problem is underconstrained, for there are an infinite number of possible three-dimensional configurations that can give rise to the same two-dimensional projection. How does the visual system select from this infinite range of possible percepts to produce the single perceptual interpretation observed phenomenally? The answer to this question is of central significance to understanding the principles behind perception, for it reveals a computational strategy quite unlike anything devised by man, and certainly unlike the algorithmic decision sequences embodied in the paradigm of digital computation. The transformation observed in visual perception gives us the clearest insight into the nature of this unique computational strategy. I propose that the principles of emergence, reification, and multistability are intimately involved in this reconstruction, and that in fact these Gestalt properties are exactly the properties needed for the visual system to address the fundamental ambiguities inherent in reflected light imagery.
The principle behind the perceptual transformation can be expressed in general terms as follows. For any given visual input there is an infinite range of possible configurations of objects in the external world which could have given rise to that same stimulus. The configuration of the stimulus constrains the range of those possible perceptual interpretations to those that line up with the stimulus in the two dimensions of the retinal image. Now although each individual interpretation within that range is equally likely with respect to the stimulus, some of those perceptual alternatives are intrinsically more likely than others, in the sense that they are more typical of objects commonly found in the world. I propose that the perceptual representation has the property that the more likely structural configurations are also more stable in the perceptual representation, and therefore the procedure used by the visual system is to essentially construct or reify all possible interpretations of a visual stimulus in parallel, as constrained by the configuration of the input, and then to select from that range of possible percepts the most stable perceptual configuration by a process of emergence. In other words, perception can be viewed as the computation of the intersection of two sets of constraints, which might be called extrinsic v.s. intrinsic constraints. The extrinsic constraints are those determined by the visual stimulus, whereas the intrinsic constraints are determined by the structural stability of the percept.
Arnheim (1969) presents an insightful analysis of this concept, which can be reformulated as follows. Consider (for simplicity) just the central "Y" vertex of figure 6 A depicted in figure 6 C. Arnheim proposes that the extrinsic constraints of inverse optics can be expressed for this stimulus using a rod-and-rail analogy as shown in figure 6 D. The three rods, representing the three edges in the visual input, are constrained in two dimensions to the configuration seen in the input, but are free to slide in depth along four rails. The rods must be elastic between their end-points, so that they can expand and contract in length. By sliding along the rails, the rods can take on any of the infinite three-dimensional configurations corresponding to the two-dimensional input of figure 6 C. For example the final percept could theoretically range from a percept of a convex vertex protruding from the depth of the page, to a concave vertex intruding into the depth of the page, with a continuum of intermediate perceptual states between these limits. There are other possibilities beyond these, for example percepts where each of the three rods is at a different depth and therefore they do not meet in the middle of the stimulus. However these alternative perceptual states are not all equally likely to be experienced. Hochberg & Brooks (1960) showed that the final percept is the one that exhibits the greatest simplicity, or prägnanz. In the case of the vertex of figure 6 C the percept tends to appear as three rods whose ends coincide in depth at the center, and meet at a mutual right angle, defining either a concave or convex corner. This reduces the infinite range of possible configurations to two discrete perceptual states. This constraint can be expressed emergently in the rod and rail model by joining the three rods flexibly at the central vertex, and installing spring forces that tend to hold the three rods at mutual right angles at the vertex. With this mechanism in place to define the intrinsic or structural constraints, the rod-and-rail model becomes a dynamic system that slides in depth along the rails, and this system is bistable between a concave and convex right angled percept, as observed phenomenally in figure 6 C. Although this model reveals the dynamic interaction between intrinsic and extrinsic constraints, this particular analogy is hard-wired to modeling the percept of the triangular vertex of figure 6 C. I will now develop a more general model that operates on this same dynamic principle, but is designed to handle arbitrary input patterns.
For the perceptual representation I propose (Lehar 2003) a volumetric block or matrix of dynamic computational elements, as suggested in figure 7 A, each of which can exist in one of two states, transparent or opaque, with opaque state units being active at all points in the volume of perceptual space where a colored surface is experienced. In other words upon viewing a stimulus like figure 6 A, the perceptual representation of this stimulus is modeled as a three-dimensional pattern of opaque state units embedded in the volume of the perceptual matrix in exactly the configuration observed in the subjective perceptual experience when viewing figure 6 A, i.e. with opaque-state elements at all points in the volumetric space that are within a perceived surface in three dimensions, as suggested in figure 6 B. All other elements in the block are in the transparent state to represent the experience of the spatial void within which perceived objects are perceived to be embedded. More generally opaque state elements should also encode the subjective dimensions of color, i.e. hue, intensity, and saturation, and intermediate states between transparent and opaque would be required to account for the perception of semi-transparent surfaces, although for now, the discussion will be limited to two states and the monochromatic case. The transformation of perception can now be defined as the turning on of the appropriate pattern of elements in this volumetric representation in response to the visual input, in order to replicate the three-dimensional configuration of surfaces experienced in the subjective percept.
The perceived surfaces due to a stimulus like 6 A appear to span the structure of the percept defined by the edges in the stimulus, somewhat like a milky bubble surface clinging to a cubical wire frame. Although the featureless portions of the stimulus between the visual edges offer no explicit visual information, a continuous surface is perceived within those regions, as well as across the white background behind the block figure, with a specific depth and surface orientation value encoded explicitly at each point in the percept. This three-dimensional surface interpolation function can be expressed in the perceptual model by assigning every element in the opaque state a surface orientation value in three dimensions, and by defining a dynamic interaction between opaque state units to fill in the region between them with a continuous surface percept. In order to express this process as an emergent one, the dynamics of this surface interpolation function must be defined in terms of local field-like forces analogous to the local forces of surface tension active at any point in a soap bubble. Figure 7 C depicts an opaque state unit representing a local portion of a perceived surface at a specific three-dimensional location and with a specific surface orientation. The planar field of this element, depicted somewhat like a planetary ring in figure 7 C, represents both the perceived surface represented by this element, as well as a field-like influence propagated by that element to adjacent units. This planar field fades smoothly with distance from the center with a Gaussian function. The effect of this field is to recruit adjacent elements within that field of influence to take on a similar state, i.e. to induce transparent state units to switch to the opaque state, and opaque state units to rotate towards a similar surface orientation value. The final state and orientation taken on by any element is computed as a spatial average or weighted sum of the states of neighboring units as communicated through their planar fields of influence, i.e. with the greatest influence from nearby opaque elements in the matrix. The influence is reciprocal between neighboring elements, thereby defining a circular relation as suggested by the principle of emergence. In order to prevent runaway positive feedback and uncontrolled propagation of surface signal, an inhibitory dynamic is also incorporated in order to suppress surface formation out of the plane of the emergent surface, by endowing the local field of each unit with an inhibitory field in order to suppress the opaque state in neighboring elements in all directions outside of the plane of its local field. The mathematical specification of the local field of influence between opaque state units is outlined in greater detail in the appendix. However the intent of the model is expressed more naturally in the global properties as described here, so the details of the local field influences are presented as only one possible implementation of the concept, provided in order to ground this somewhat nebulous idea in more concrete terms.
The global properties of the system should be such that if the elements in the matrix were initially assigned randomly to either the transparent or opaque state, with random surface orientations for opaque-state units, the mutual field-like influences would tend to amplify any group of opaque-state elements whose planar fields happened to be aligned in an approximate plane, and as that plane of active units feeds back on its own activation, the orientations of its elements would conform ever closer to that of the plane, while elements outside of the plane would be suppressed to the transparent state. This would result in the emergence of a single plane of opaque-state units as a dynamic global pattern of activation embedded in the volume of the matrix, and that surface would be able to flex and stretch much like a bubble surface, although unlike a real bubble, this surface is defined not as a physical membrane, but as a dynamic sheet of active elements embedded in the matrix. This volumetric surface interpolation function will now serve as the backdrop for an emergent reconstruction of the spatial percept around a three-dimensional skeleton or framework constructed on the basis of the visual edges in the scene.
A visual edge can be perceived as an object in its own right, like a thin rod or wire surrounded by empty space. More often however an edge is seen as a discontinuity in a surface, either as a corner or fold, or perhaps as an occlusion edge like the outer perimeter of a flat figure viewed against a more distant background. The interaction between a visual edge and a perceived surface can therefore be modeled as follows. The two-dimensional edge from the retinal stimulus projects a different kind of field of influence into the depth dimension of the volumetric matrix, as suggested by the gray shading in figure 7 A, to represent the three-dimensional locus of all possible edges that project to the two-dimensional edge in the image. In other words, this field expresses the inverse optics probability field or extrinsic constraint due to a single visual edge. Wherever this field intersects opaque-state elements in the volume of the matrix, it changes the shape of their local fields of influence from a coplanar interaction to an orthogonal, or corner interaction as suggested by the local force field in figure 7 D. The corner of this field should align parallel to the visual edge, but otherwise remain unconstrained in orientation except by interactions with adjacent opaque units. Visual edges can also denote occlusion, and so opaque-state elements can also exist in an occlusion state, with a coplanarity interaction in one direction only, as suggested by the occlusion field in figure 7 E. Therefore, in the presence of a single visual edge, a local element in the opaque state should have an equal probability of changing into the orthogonality or occlusion state, with the orthogonal or occlusion edge aligned parallel to the inducing visual edge. Elements in the orthogonal state tend to promote orthogonality in adjacent elements along the perceived corner, while elements in the occlusion state promote occlusion along that edge. In other words, an edge will tend to be perceived as a corner or occlusion percept along its entire length, although the whole edge may change state back and forth as a unit in a multistable manner. The appendix presents a more detailed mathematical description of how these orthogonality and occlusion fields might be defined. The presence of the visual edge in figure 7 A therefore tends to crease or break the perceived surface into one of the different possible configurations shown in figure 8 A through D. The final configuration selected by the system would depend not only on the local image region depicted in figure 8, but also on forces from adjacent regions of the image, in order to fuse the orthogonal or occlusion state elements seamlessly into nearby coplanar surface percepts.
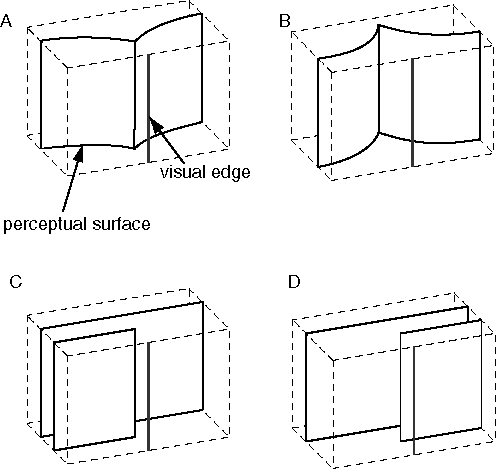
Visual illusions like the Kanizsa figure shown in figure 4 A suggest that edges in a stimulus that are in a collinear configuration tend to link up in perceptual space to define a larger global edge connecting the local edges. This kind of collinear boundary completion is expressed in this model as a physical process analogous to the propagation of a crack or fold in a physical medium. A visual edge which fades gradually produces a crease in the perceptual medium that tends to propagate outward beyond the edge as suggested in figure 9 A. If two such edges are found in a collinear configuration, the perceptual surface will tend to crease or fold between them as suggested in figure 9 B. This tendency is accentuated if additional evidence from adjacent regions support this configuration. This can be seen in figure 9 C where fading horizontal lines are seen to link up across the figure to create a percept of a folded surface in depth, which would otherwise appear as a regular hexagon, as seen in figure 9 D.
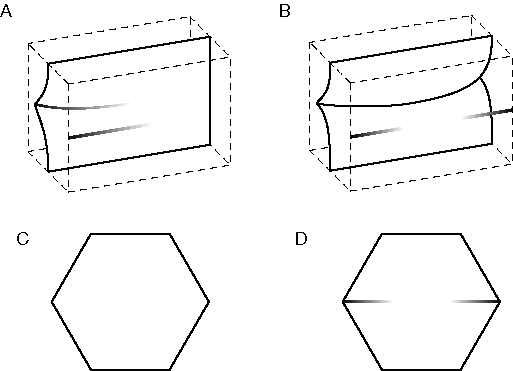
Gestalt theory emphasized the significance of closure as a prominant factor in perceptual segmentation, since an enclosed contour is seen to promote a figure / ground segregation (Koffka 1935 p. 178). For example an outline square tends to be seen as a square surface in front of a background surface that is complete and continuous behind the square, as suggested in the perceptual model depicted in figure 10 A. The problem is that closure is a "gestaltqualität", a quality defined by a global configuration that is difficult to specify in terms of any local featural requirements, especially in the case of irregular or fragmented contours as seen in figure 10 B. In this model an enclosed contour breaks away a piece of the perceptual surface, completing the background amodally behind the occluding foreground figure. In the presence of irregular or fragmented edges the influence of the individual edge fragments act collectively to break the perceptual surface along that contour as suggested in figure 10 C, like the breaking of a physical surface that is weakened along an irregular line of cracks or holes. The final scission of figure from ground is therefore driven not so much by the exact path of the individual irregular edges, as it is by the global configuration of the emergent gestalt.
In the case of vertices or intersections between visual edges, the different edges interact with one another favoring the percept of a single vertex at that point. For example the three edges defining the three-way "Y" vertex shown in figure 6 C promote the percept of a single three-dimensional corner, whose depth profile depends on whether the corner is perceived as convex or concave. In the case of figure 6 A, the cubical percept constrains the central "Y" vertex as a convex rather than a concave trihedral percept. I propose that this dynamic behavior can be implemented using the same kinds of local field-forces described in the appendix to promote mutually orthogonal completion in three dimensions, wherever visual edges meet at an angle in two dimensions. Figure 11 A depicts the three-dimensional influence of the two-dimensional Y-vertex when projected on the front face of the volumetric matrix. Each plane of this three-planed structure promotes the emergence of a corner or occlusion percept at some depth within that plane. But the effects due to these individual edges are not independent. Consider for example, first the vertical edge projecting from the bottom of the vertex. By itself, this edge might produce a folded percept as suggested in figure 11 B, which could occur through a range of depths, and a variety of orientations in depth, and in concave or convex form. But the two angled planes of this percept each intersect the other two fields of influence due to the other two edges of the stimulus, as suggested in figure 11 B, thus favoring the emergence of those edges' perceptual folds at that same depth, resulting in a single trihedral percept at some depth in the volumetric matrix, as suggested in figure 11 C. Any dimension of this percept that is not explicitly specified or constrained by the visual input, remains unconstrained. In other words, the trihedral percept is embedded in the volumetric matrix in such a way that its three component corner percepts are free to slide inward or outward in depth, to rotate through a small range of angles, and to flip in bistable manner between a convex and concave trihedral configuration. The model now expresses the multistability of the rod-and-rail analogy shown in figure 6 D, but in a more generalized form that is no longer hard-wired to the Y-vertex input shown in figure 6 C, but can accommodate any arbitrary configuration of lines in the input image. A local visual feature like an isolated Y-vertex generally exhibits a larger number of stable states, whereas in the context of adjacent features the number of stable solutions is often diminished. This explains why the cubical percept of figure 6 A is stable, while its central Y-vertex alone as shown in figure 6 C is bistable. The fundamental multistability of figure 6 A can be revealed by the addition of a different spatial context, as depicted in figure 11 D.

Perspective cues offer another example of a computation that is inordinately complicated in most models. However in a fully reified spatial model perspective can be computed relatively easily with only a small change in the geometry of the model. Figure 12 A shows a trapezoid stimulus, which has a tendency to be perceived in depth, i.e. the shorter top side tends to be perceived as being the same length as the longer base, but apparently diminished by perspective. Arnheim (1969) suggests a simple distortion to the volumetric model to account for this phenomenon, which can be reformulated as follows. The height and width of the volumetric matrix are diminished as a function of depth, as suggested in figure 12 B, transforming the block shape into a truncated pyramid that tapers in depth. The vertical and horizontal dimensions represented by that space however are not diminished, in other words, the larger front face and the smaller rear face of the volumetric structure represent equal areas in perceived space, by unequal areas in representational space, as suggested by the converging grid lines in the figure. All of the spatial interactions described above, for example the collinear propagation of corner and occlusion percepts, would be similarly distorted in this space. Even the angular measure of orthogonality is distorted somewhat by this transformation. For example the perceived cube depicted in the solid volume of figure 12 B is metrically shrunken in height and width as a function of depth, but since this shrinking is in the same proportion as the shrinking of the space itself, the depicted irregular cube represents a percept of a regular cube with equal sides and orthogonal faces. The propagation of the field of influence in depth due to a two-dimensional visual input on the other hand does not shrink with depth. A projection of the trapezoid of figure 12 A would occur in this model as depicted in figure 12 C, projecting the trapezoidal form backward in parallel, independent of the convergence of the space around it. The shaded surfaces in figure 12 C therefore represent the locus of all possible spatial interpretations of the two-dimensional trapezoid stimulus of figure 12 A, or the extrinsic constraints for the spatial percept due to this stimulus. For example one possible perceptual interpretation is of a trapezoid parallel to the plane of the page, which can be perceived to be either nearer or farther in depth, but since the size scale shrinks as a function of depth, the percept will be experienced as larger in absolute size (as measured against the shrunken spatial scale) when perceived as farther away, and as smaller in absolute size (as measured against the expanded scale) when perceived to be closer in depth. This corresponds to the phenomenon known as Emmert's Law (Coren et al. 1994), whereby a retinal after-image appears larger when viewed against a distant background than when viewed against a nearer background. Now there are also an infinite number of alternative perceptual interpretations of the trapezoidal stimulus, some of which are depicted by the dark shaded lines of figure 12 D. Most of these alternative percepts are geometrically irregular, representing figures with unequal sides and odd angles. But of all these possibilities, there is one special case, depicted in black lines in figure 12 D, in which the convergence of the sides of the perceived form happens to coincide exactly with the convergence of the space itself. In other words, this particular percept represents a regular rectangle viewed in perspective, with parallel sides and right angled corners, whose nearer (bottom) and farther (top) horizontal edges are the same length in the distorted perceptual space. While this rectangular percept represents the most stable interpretation, other possible interpretations might be suggested by different contexts. The most significant feature of this concept of perceptual processing is that the result of the computation is expressed not in the form of abstract variables encoding the depth and slope of the perceived rectangle, but in the form of an explicit three-dimensional replica of the surface as it is perceived to exist in the world.
An explicit volumetric representation of perceived space as proposed
here must necessarily be bounded in some way in order to allow a
finite representational space to map to the infinity of external
space, as suggested in figure 2. The nonlinear compression of the
depth dimension observed in phenomenal space can be modeled
mathematically with a vergence measure, which maps the infinity of
Euclidean distance into a finite bounded range, as suggested in figure
13 A.This produces a representation reminiscent of museum diaramas,
like the one depicted in figure 13 B, where objects in the foreground
are represented in full depth, but the depth dimension gets
increasingly compressed with distance from the viewer, eventually
collapsing into a flat plane corresponding to the background. This
vergence measure is presented here merely as a nonlinear compression
of depth in a monocular spatial representation, as opposed to a real
vergence value measured in a binocular system, although this system
could of course serve both purposes in biological vision. Assuming
unit separation between the eyes in a binocular system, this
compression is defined by the equation where n is the vergence measure of
depth, and r is the Euclidean range, or distance in
depth. Actually, since vergence is large at short range and smaller
at long range, it is actually the "p-compliment" vergence measure r that is used in the representation, where
r = (p-n), and r ranges from
0 at r = 0, to p at r =
infinity.
What does this kind of compression mean in an isomorphic
representation? If the perceptual frame of reference is compressed
along with the objects in that space, then the compression need not be
perceptually apparent. Figure 13 C depicts this kind of compressed
reference grid. The unequal intervals between adjacent grid lines in
depth define intervals that are perceived to be of equal length, so
the flattened cubes defined by the distorted grid would appear
perceptually as regular cubes, of equal height, breadth, and
depth. This compression of the reference grid to match the compression
of space would, in a mathematical system with infinite resolution,
completely conceal the compression from the percipient. In a real
physical implementation there are two effects of this compression that
would remain apparent perceptually, due to the fact that the spatial
matrix itself would have to have a finite perceptual resolution. The
resolution of depth within this space is reduced as a function of
depth, and beyond a certain limiting depth, all objects are perceived
to be flattened into two dimensions, with zero extent in depth. This
phenomenon is observed perceptually, where the sun, moon, and distant
mountains appear as if they are pasted against the flat dome of the
sky. The other two dimensions of space can also be bounded by
converting the x and y of Euclidean space into azimuth
and elevation angles, a and b, producing an angle / angle / vergence
representation, as shown in figure 14 A. Mathematically this
transformation converts the point P(a,b,r) in polar coordinates to point Q(a,b,r) in this bounded spherical representation. In
other words, azimuth and elevation angles are preserved by this
transformation while the radial distance in depth r is
compressed to the vergence representation r
as described above. This spherical coordinate system has the
ecological advantage that the space near the body is represented at
the highest spatial resolution, whereas the less important more
distant parts of space are represented at lower resolution. All depths
beyond a certain radial distance are mapped to the surface of the
representation which corresponds to perceptual infinity.
The mathematical form of this distortion is depicted in figure 14 B,
where the distorted grid depicts the perceptual representation of an
infinite Cartesian grid with horizontal and vertical grid lines spaced
at equal intervals. This geometrical transformation from the infinite
Cartesian grid actually represents a unique kind of perspective
transformation on the Cartesian grid. In other words, the transformed
space looks like a perspective view of a Cartesian grid when viewed
from inside, with all parallel lines converging to a point in opposite
directions. The significance of this observation is that by mapping
space into a perspective-distorted grid, the distortion of perspective
is removed, in the same way that plotting log data on a log plot
removes the logarithmic component of the data. Figure 14 C shows how
this space would represent the perceptual experience of a man walking
down a road. If the distorted reference grid of figure 14 B is used to
measure lines and distances in figure 14 C, the bowed line of the road
on which the man is walking is aligned with the bowed reference grid
and therefore is perceived to be straight. Therefore the distortion
of straight lines into curves in the perceptual representation is not
immediately apparent to the percipient, because they are perceived to
be straight. However in a global sense there are peculiar distortions
that are apparent to the percipient caused by this deformation of
Euclidean space. For while the sides of the road are perceived to be
parallel, they are also perceived to meet at a point on the
horizon. The fact that two lines can be perceived to be both straight
and parallel and yet to converge to a point both in front and behind
the percipient indicates that our internal representation itself must
be curved. The proposed representation of space has exactly this
property. Parallel lines do not extend to infinity but meet at a point
beyond which they are no longer represented. Likewise the vertical
walls of the houses in figure 14 C bow outwards away from the
observer, but in doing so they follow the curvature of the reference
lines in the grid of figure 14 B, and are therefore perceived as being
both straight, and vertical. Since curved lines in this spherical
representation represent straight lines in external space, all of the
spatial interactions discussed in the previous section, including the
coplanar interactions, and collinear creasing of perceived surfaces,
must follow the grain or curvature of collinearity defined within this
distorted coordinate system. The distance scale encoded in the grid of
figure 14 B replaces the regularly spaced Cartesian grid by a
nonlinear collapsing grid whose intervals are spaced ever closer as
they approach perceptual infinity but nevertheless represent equal
intervals in external space. This nonlinear collapsing scale thereby
provides an objective measure of distance in the perspective-distorted
perceptual world. For example the houses in figure 14 C would be
perceived to be approximately the same size and depth, although the
farther house is experienced at a lower perceptual resolution.
Figure 14 D depicts how a slice of Euclidean space of fixed height and
width would appear in the perceptual sphere, extending to perceptual
infinity in one direction, like a slice cut from the spherical
representation of figure 14 C. This slice is similar to the truncated
pyramid shape shown in figure 12 B, with the difference that the
horizontal and vertical scale of representational space diminishes in
a nonlinear fashion as a function of distance in depth. In other
words, the sides of the pyramid in figure 14 B converge in curves
rather than in straight lines, and the pyramid is no longer truncated,
but extends in depth all the way to the vanishing point at
representational infinity. An input image is projected into this
spherical space using the same principles as before.
One of the most disturbing properties of the phenomenal world for
models of the perceptual mechanism involves the subjective impression
that the phenomenal world rotates relative to our perceived head as
our head turns relative to the world, and that objects in perception
are observed to translate and rotate while maintaining their perceived
structural integrity and recognized identity. This suggests that the
internal representation of external objects and surfaces is not
anchored to the tissue of the brain, as suggested by current concepts
of neural representation, but that perceptual structures are free to
rotate and translate coherently relative to the neural substrate, as
suggested in Köhler's field theory (Köhler & Held 1947). This
issue of brain anchoring is so troublesome that it is often cited as a
counter-argument for an isomorphic representation, since it is
difficult to conceive of the solid spatial percept of the surrounding
world having to be reconstructed anew in all its rich spatial detail
with every turn of the head (Gibson 1979, O'Regan 1992). However an
argument can be made for the adaptive value of a neural representation
of the external world that could break free of the tissue of the
sensory or cortical surface in order to lock on to the more meaningful
coordinates of the external world, if only a plausible mechanism could
be conceived to achieve this useful property.
Even in the absence of a neural model with the required properties,
the invariance property can be encoded in a perceptual model. In the
case of rotation invariance, this property can be quantified by
proposing that the spatial structure of a perceived object and its
orientation are encoded as separable variables. This would allow the
structural representation to be updated progressively from successive
views of an object that is rotating through a range of
orientations. However the rotation invariance property does not mean
that the encoded form has no defined orientation, but rather that the
perceived form is presented to consciousness at the orientation and
rate of rotation that the external object is currently perceived to
possess. In other words, when viewing a rotating object, like a person
doing a cartwheel, or a skater spinning about her vertical axis, every
part of that visual stimulus is used to update the corresponding part
of the internal percept even as that percept rotates within the
perceptual manifold to remain in synchrony with the rotation of the
external object. The perceptual model need not explain how this
invariance is achieved neurophysiologically, it must merely express
the invariance property computationally, regardless of the "neural
plausibility" or computational efficiency of that calculation. For
the perceptual model is more a quantitative description of the
phenomenon rather than a theory of neurocomputation. The property of
translation invariance can be similarly quantified in the
representation by proposing that the structural representation can be
calculated from a stimulus that is translating across the sensory
surface, to update a perceptual effigy that translates with respect to
the representational manifold, while maintaining its structural
integrity. This accounts for the structural constancy of the perceived
world as it scrolls past a percipient walking through a scene, with
each element of that scene following the proper curved perspective
lines as depicted in figure 2, expanding outwards from a point up
ahead, and collapsing back to a point behind, as would be seen in a
cartoon movie rendition of figure 2.
The fundamental invariance of such a representation offers an
explanation for another property of visual perception, i.e. the way
that the individual impressions left by each visual saccade are
observed to appear phenomenally at the appropriate location within the
global framework of visual space depending on the direction of
gaze. This property can be quantified in the perceptual model as
follows. The two-dimensional image from the spherical surface of the
retina is copied onto a spherical surface in front of the eyeball of
the perceptual effigy, from whence the image is projected radially
outwards in an expanding cone into the depth dimension of the internal
perceptual world as suggested in figure 15, as an inverse analog of
the cone of light received from the world by the eye. Eye, head, and
body orientation relative to the external world are taken into account
in order to direct the visual projection of the retinal image into the
appropriate sector of perceived space, as determined from
proprioceptive and kinesthetic sensations in order to update the image
of the body configuration relative to external space. The percept of
the surrounding environment therefore serves as a kind of
three-dimensional frame buffer expressed in global coordinates, that
accumulates the information gathered in successive visual saccades and
maintains an image of that external environment in the proper
orientation relative to a spatial model of the body, compensating for
body rotations or translations through the world. Portions of the
environment that have not been updated recently gradually fade from
perceptual memory, which is why it is easy to bump one's head after
bending for some time under an overhanging shelf, or why it is
possible to advance only a few steps safely after closing one's eyes
while walking.
The picture of visual processing revealed by the phenomenological
approach is radically different from the picture revealed by
neurophysiological studies. In fact, the computational transformations
observed phenomenologically are implausible in terms of contemporary
concepts of neurocomputation and even in terms of computer
algorithms. However the history of psychology is replete with examples
of plausibility arguments based on the limited technology of the time
which were later invalidated by the emergence of new technologies. The
outstanding achievements of modern technology, especially in the field
of information processing systems, might seem to justify our
confidence to judge the plausibility of proposed processing
algorithms. And yet, despite the remarkable capabilities of modern
computers, there remain certain classes of problems that appear to be
fundamentally beyond the capacity of the digital computer. In fact the
very problems that are most difficult for computers to address, such
as extraction of spatial structure from a visual scene especially in
the presence of attached shadows, cast shadows, specular reflections,
occlusions, perspective distortions, as well as the problems of
navigation in a natural environment, etc. are problems that are
routinely handled by biological vision systems, even those of simpler
animals. On the other hand, the kinds of problems that are easily
solved by computers, such as perfect recall of vast quantities of
meaningless data, perfect memory over indefinite periods, detection of
the tiniest variation in otherwise identical data, exact repeatability
of even the most complex computations, are the kinds of problems that
are inordinately difficult for biological intelligence, even that of
the most complex of animals. It is therefore safe to assume that the
computational principles of biological vision are fundamentally
different from those of digital computation, and therefore
plausibility arguments predicated on contemporary concepts of what is
computable are not applicable to biological vision. If we allow that
our contemporary concepts of neurocomputation are so embryonic that
they should not restrict our observations of the phenomenal properties
of perception, the evidence for a Gestalt Bubble model of perceptual
processing becomes overwhelming.
The phenomena of hallucinations and dreams demonstrate that the mind
is capable of generating complete spatial percepts of the world,
including a percept of the body and the space around it (Revonsuo
1995). It is unlikely that this remarkable capacity is used only to
create such illusory percepts. More likely, dreams and hallucinations
reveal the capabilities of an imaging system that is normally driven
by the sensory input, generating perceptual constructs that are
coupled to external reality.
Studies of mental imagery (Kosslyn 1980, 1994) have characterized the
properties of this imaging capacity, and confirmed the
three-dimensional nature of the encoding and processing of mental
imagery. Pinker (1980) shows that the scanning time between objects
in a remembered three-dimensional scene increases linearly with
increasing distance between objects in three dimensions. Shepard &
Metzler. (1971) show that the time for rotation of mental images is
proportional to the angle through which they are rotated. Kosslyn
shows that it takes time to expand the size of mental images, and that
smaller mental images are more difficult to scrutinize (Kosslyn
1975). As unexpected as these findings may seem for theorists of
neural representation, they are perfectly consistent with the
subjective experience of mental imagery. On the basis of these
findings, Pinker (1988) derived a volumetric spatial medium to account
for the observed properties of mental image manipulation which is very
similar to the model proposed here, i.e. with a volumetric
azimuth/elevation coordinate system that is addressable both in
subjective viewer-centered, and objective viewer-independent
coordinates, and with a compressive depth scale. The phenomenon of hemi-neglect (McFie & Zangwill 1960, Heilman &
Watson 1977, Heilman et al. 1985, Kolb & Whishaw 1996) reveals the
effects of damage to the spatial representation, destroying the
capacity to represent spatial percepts in one half of phenomenal
space. Such patients are not simply blind to objects to one side, but
are blind to the very existence of a space in that direction as a
potential holder of objects. For example, neglect patients will
typically eat food only from the right half of their plate, and
express surprise at the unexpected appearance of more food when their
plate is rotated 180 degrees. This condition even persists when the
patient is cognitively aware of their deficit (Sacks 1985). Bisiach
et al. (1978,1981) show how this condition can also impair
mental imaging ability. They describe a neglect patient who, when
instructed to recall a familiar scene viewed from a certain direction,
can recall only objects from the right half of his remembered
space. When instructed to mentally turn around and face in the
opposite direction, the patient now recalls only objects from the
other side of the scene, that now fall in the right half of his mental
image space. The condition of hemi-neglect therefore suggests damage
to the left half of a three-dimensional imaging mechanism that is used
both for perception and for the generation of mental imagery. Note
that hemi-neglect also includes a neglect of the left side of the
body, which is consistent with the fact that the body percept is
included as an integral part of the perceptual
representation.
The condition of hemi-neglect initially caused a great stir in
psychological circles because it appeared to be concrete evidence for
an explicit spatial representation in the brain (Denny-Brown &
Chambers 1958, de Renzi 1982, Bisiach & Luzzatti 1978, Bisiach et
al. 1981). It is curious that half of phenomenal space should have to
disappear for psychologists to take account of its existence in the
first place! But after the initial excitement, the naïve realists
quickly marshalled their defenses with an array of arguments which
many believe to dispose of the troublesome issue of hemi-neglect. Some
argue that hemi-neglect is not a failure of spatial representation,
but rather an imbalance of attention, or `orienting response',
i.e. that half of phenomenal space does not actually disappear, but
that the neglect patient is merely inclined to ignore its
presence. (Heilman & Watson 1977, Heilman et al. 1985, Kinsbourne
1987, 1993) But even if these arguments are valid, they do not account
for the presence in visual consciousness of the spatial structure of
the phenomenal world whenever it is not being ignored or neglected;
they merely offer a convenient escape clause to make neglect syndrome
seem no more mysterious than normal spatial perception. Others argue
that the phenomenon of hemi-neglect fractionates to a number of
distinct patterns of impairment (Vallar 1998 p. 88). For example many
neglect patients can describe the global gestalt of a figure, but when
copying its local features, leave those on the left side out (Marshall
& Halligan 1995). Present accounts of the multiple forms of neglect
refer to several spatial maps and their interaction (e.g. Ladavas et
al. 1997). This highlights a conflict between the phenomenal and
neurophysiological evidence, the former presenting a unified spatial
structure in visual experience, while the latter suggests discrete
mechanisms in different cortical areas. To the naive realist this
suggests that the spatial percept must be somehow illusory, which
thereby supposedly relieves neuroscience from any obligation to
account for its manifest properties. What is curious about the debate
over neglect is the passion that it engenders. The evidence presented
by each side never seems to convince the opposition, because the
debate is not really about neglect, but about its implications for
perceptual representation, and that issue is not so much a matter of
experimental evidence but of the interpretation of that evidence, or
the foundational assumptions with which one comes to the debate in the
first place. Whatever the physiological reality behind the phenomenon
of hemi-neglect, the Gestalt Bubble model offers at least a concrete
description of this otherwise paradoxical phenomenon. The idea that this spatial imaging system employs an explicit
volumetric spatial representation is suggested by the fact that
disparity tuned cells have been found in the cortex (Barlow et
al. 1967), as predicted by the Projection Field Theory of
binocular vision (Kaufman 1974, Boring 1933, Charnwood 1951, Marr &
Poggio 1976, Julesz 1971), which is itself a volumetric
model. Psychophysical evidence for a volumetric representation comes
from the fact that perceived objects in depth exhibit attraction and
repulsion in depth (Westheimer & Levi 1987, Mitchison 1993) in a
manner that is suggestive of a short-range attraction and longer-range
repulsion in depth, analogous to the center-surround processing in the
retina. Brookes & Stevens (1989) discuss the analogy between
brightness and depth perception, and show that a number of brightness
illusions that have been attributed to such center-surround processing
have corresponding illusions in depth. Similarly, Anstis & Howard
(1978) have demonstrated a Craik-O'Brien-Cornsweet illusion in depth
by cutting the near surface of a block of wood with a depth profile
matching the brightness cusp of the brightness illusion, resulting in
an illusory percept of a difference in depth of the surfaces on either
side of the cusp. As in the brightness illusion, therefore, the depth
difference at the cusp appears to propagate a perceptual influence out
to the ends of the block, suggesting a spatial diffusion of depth
percept between depth edges.
The many manifestations of constancy in perception have always posed a
serious challenge for theories of perception because they reveal that
the percept exhibits properties of the distal object rather than the
proximal stimulus, or pattern of stimulation on the sensory
surface. The Gestalt Bubble model explains this by the fact that the
information encoded in the internal perceptual representation itself
reflects the properties of the distal object rather than the proximal
stimulus. Size constancy is explained by the fact that objects
perceived to be more distant are represented closer to the outer
surface of the perceptual sphere, where the collapsing reference grid
corrects for the shrinkage of the retinal image due to perspective. An
object perceived to be receding in depth therefore is expected
perceptually to shrink in retinal size along with the shrinking of the
grid in depth, and conversely, shrinking objects tend to be perceived
as receding. Rock & Brosgole. (1964), show that perceptual grouping by
proximity is determined not by proximity in the two-dimensional
retinal projection of the figure, but rather by the three-dimensional
perceptual interpretation. A similar finding is shown by Green & Odum
(1986). Shape constancy is exemplified by the fact that a rectangle
seen in perspective is not perceived as a trapezoid, as its retinal
image would suggest. The Müller-Lyer and Ponzo illusions are
explained in similar fashion (Tausch 1954, Gregory 1963, Gillam 1971,
1980), the converging lines in those figures suggesting a surface
sloping in depth, so that features near the converging ends are
measured against a more compressed reference grid than the
corresponding feature near the diverging ends of those lines.
Several researchers have presented psychophysical evidence for a
spatial interpolation in depth, which is difficult to account for
except with a volumetric representation in which the interpolation is
computed explicitly in depth (Attneave 1982). Kellman et al. (1996)
have demonstrated a coplanar completion of perceived surfaces in depth
in a manner analogous to the collinear completion in the Kanizsa
figure. Barrow & Tenenbaum (1981, p. 94 and Figure 6.1) show how a
two-dimensional wire-frame outline held in front of a dynamic random
noise pattern stimulates a three-dimensional surface percept spanning
the outline like a soap film, and that perceived surface undergoes a
Necker reversal together with the reversal of the perimeter wire. Ware
& Kennedy (1978) have shown that a three-dimensional rendition of the
Ehrenstein illusion constructed of a set of rods converging on a
circular hole, creates a three-dimensional version of the illusion
that is perceived as a spatial structure in depth, even when rotated
out of the fronto-parallel plane, complete with a perception of
brightness at the center of the figure. This illusory percept appears
to hang in space like a faintly glowing disk in depth, reminiscent of
the neon color spreading phenomenon. A similar effect can be achieved
with a three-dimensional rendition of the Kanizsa figure. If the
Ehrenstein and Kanizsa figures are explained by spatial interpolation
in models such as Grossberg & Mingolla (1985), then the corresponding
three-dimensional versions of these illusions must involve a
volumetric computational matrix to perform the interpolation in depth.
Collett (1985) has investigated the interaction between monocular and
binocular perception using stereoscopically presented line drawings in
which some features are presented only monocularly, i.e. their depth
information is unspecified. Collett shows that such features tend to
appear perceptually at the same depth as adjacent binocularly
specified features, as if under the influence of an attractive force
in depth generated by the binocular feature. In ambiguous cases the
percept is often multi-stable, jumping back and forth in depth,
especially when monocular perspective cues conflict with the binocular
disparity information. The perceived depth of the monocularly
specified surfaces is measured psychophysically using a
three-dimensional disparity-specified cursor, whose depth is adjusted
by the subject to match the depth of the perceived surface at that
point. Subjects report a curious interaction between the cursor and
the perceived surface, which is observed to flex in depth towards the
cursor at small disparity differences, in the manner of the attraction
and repulsion in depth reported by Westheimer & Levi (1987). This
dynamic influence is suggestive of a grouping by proximity mechanism,
expressed as a field-like attraction between perceived features in
depth, and the flexing of the perceived surface near the 3-D cursor,
as well as the multistability in the presence of conflicting
perspective and disparity cues, are suggestive of a Gestalt Bubble
model.
Carman & Welch (1992) employ a similar cursor to measure the
perceived depth of three-dimensional illusory surfaces seen in Kanizsa
figure stereograms, whose inducing edges are tilted in depth in a
variety of configurations, as shown in Figure 16 A. Note how the
illusory surface completes in depth by coplanar interpolation defining
a smooth curving surface. The subjects in this experiment also
reported a flexing of the perceived surface in depth near the
disparity-defined cursor. Equally interesting is the "port hole"
illusion seen in the reverse-disparity version of this figure, where
the circular completion of the port holes generates an ambiguous
unstable semi-transparent percept at the center of the figure that is
characteristic of the Gestalt Bubble model. Kellman & Shipley (1991)
and Idesawa (1991) report the emergence of more complex illusory
surfaces in depth, using similar illusory stereogram stimuli as shown
in Figure 16 B and C. It is difficult to deny the reality of a precise
high-resolution spatial interpolation mechanism in the face of these
compelling illusory percepts. Whatever the neurophysiological basis of
these phenomena, the Gestalt Bubble model offers a mathematical
framework for a precise description of the information encoded in
these elaborate spatial percepts, independent of the confounding
factor of neurophysiological considerations.
The sophistication of the perceptual reification capacity is revealed
by the apparent motion phenomenon (Coren et al. 1994) which, in its
simplest form consists of a pair of alternately flashing lights, that
generates a percept of a single light moving back and forth between
the flashing stimuli. With more complex variations of the stimulus,
the illusory percept is observed to change color or shape in
mid-flight, to carry illusory contours, or to carry a texture region
bounded by an illusory contour between the alternately flashing
stimuli (Coren et al. 1994). Most pertinent to the discussion of a
spatial representation is the fact that the illusory percept is
observed to make excursions into the third dimension when that
produces a simpler percept. For example if an obstacle is placed
between the flashing stimuli so as to block the path between them, the
percept is observed to pass either in front of, or behind the obstacle
in depth. Similarly, if the two flashing stimuli are in the shape of
angular features like a "<" and ">" shape, this angle is observed to
rotate in depth between the flashing stimuli, preserving a percept of
a rigid rotation in depth, in preference to a morphological
deformation in two dimensions. The fact that the percept transitions
so readily into depth suggests the fundamental nature of the depth
dimension for perception.
While the apparent motion effects reify whole perceptual gestalts, the
elements of this reification, such as the field-like diffusion of
perceived surface properties, are seen in such diverse phenomena as
the perceptual filling-in of the Kanizsa figure (Takeichi et
al. 1992), the Craik-O'Brien-Cornsweet effect (Cornsweet 1970), the
neon color spreading effect (Bressan 1993), the filling-in of the
blind spot (Ramachandran 1992), color bleeding due to retinal
stabilization (Heckenmuller 1965, Yarbus 1967), the motion capture
effect (Ramachandran & Anstis 1986), and the aperture problem in
motion perception (Movshon et al. 1986). In all of these phenomena, a
perceived surface property (brightness, transparency, color, motion,
etc.) is observed to spread from a localized origin, not into a fuzzy
ill-defined region, but rather, into a sharply bounded region
containing a homogeneous perceptual quality, and this filling-in
occurs as readily in depth in a perspective view as in the
frontoparallel plane. The time has come to recognize that these
phenomena do not represent exceptional or special cases, nor are they
illusory in the sense of lacking a neurophysiological
counterpart. Rather, these phenomena reveal a general principle of
neurocomputation that is ubiquitous in biological vision.
Evidence for the spherical nature of perceived space dates back to
observations by Helmholtz (1925). A subject in a dark room is
presented with a horizontal line of point-lights at eye level in the
frontoparallel plane, and instructed to adjust their displacement in
depth, one by one, until they are perceived to lie in a straight line
in depth. The result is a line of lights that curves inwards towards
the observer, the amount of curvature being a function of the distance
of the line of lights from the observer. Helmholtz recognized this
phenomenon as evidence of the non-Euclidean nature of perceived
space. The Hillebrand-Blumenfeld alley experiments (Hillebrand 1902,
Blumenfeld 1913) extended this work with different configurations of
lights, and mathematical analysis of the results (Luneburg 1950, Blank
1958) characterized the nature of perceived space as Riemannian with
constant Gaussian curvature (see Graham 1965, Foley 1978, and Indow
1991 for a review). In other words, perceived space bows outward from
the observer, with the greatest distortion observed proximal to the
body, as suggested by the Gestalt Bubble model. Heelan (1983) presents
a more modern formulation of the hyperbolic model of perceived space,
and provides further supporting evidence from art and illusion.
It is perhaps too early to say definitively whether the model
presented here can be formulated to address all of the phenomena
outlined above. What is becoming increasingly clear however is the
inadequacy of the conventional feed-forward abstraction approach to
account for these phenomena, and that therefore novel and
unconventional approaches to the problem should be given serious
consideration. The general solution offered by the Gestalt Bubble
model to all of these problems in perception is that the internal
perceptual representation encodes properties of the distal object
rather than of the proximal stimulus, that the computations of spatial
perception are most easily performed in a fully spatial matrix, in a
manner consistent with the subjective experience of perception.
I have presented an elaborate model of perception that incorporates
many of the concepts and principles introduced by the original Gestalt
movement. While the actual mechanisms of the proposed model remain
somewhat vague and poorly specified, there are a number of prominent
aspects of visual experience accounted for by this approach to
modeling perception which are generally ignored by other models.
These phenomena are so immediately manifest in the subjective
experience of perception that they need hardly be tested
psychophysically. And yet curiously, these most obvious properties of
perception have been systematically ignored by neural modelers, even
though the central significance of these phenomena was highlighted
decades ago by the Gestaltists. There are two reasons why these
prominent aspects of perception have been consistently ignored. The
first results from the outstanding success of the single-cell
recording technique, which has shifted our theoretical emphasis from
field-like theories of whole aspects of perception, to point-like
theories of the elements of neural computation. Like the classical
Introspectionists, who refused to acknowledge perceptual experiences
that were inconsistent with their preconceived notions of sensory
representation, the Neuroreductionists of today refuse to consider
aspects of perception that are inconsistent with current theories of
neural computation, and some of them are even prepared to deny
consciousness itself in a heroic attempt to save the sinking paradigm.
There is another factor that has made it possible to ignore these most
salient aspects of perception, which is that perceptual entities, such
as the solid volumes and empty spaces we perceive around us, are
easily confused with real objects and spaces in the objective external
world. The illusion of perception is so compelling that we mistake the
percept of the world for the real world itself. And yet this naïve
realist view that we can somehow perceive the world directly, is
inconsistent with the physics of perception. If perception is a
consequence of neural processing of the sensory input, a percept
cannot in principle escape the confines of our head to appear in the
world around us, any more than a computation in a digital computer can
escape the confines of the computer. We cannot therefore in principle
have direct experience of objects in the world itself, but only of the
internal effigies of those objects generated by mental processes. The
world we see around us therefore can only be an elaborate, though very
compelling illusion, which must in reality correspond to perceptual
data structures and processes occurring actually within our own
head. As soon as we examine the world we see around us, not as a
physical scientist observing the physical world, but as a perceptual
scientist observing a rich and complex internal percept, only then
does the rich spatial nature of perceptual processing become
immediately apparent. It was this central insight into the illusion of
consciousness that formed the key inspiration of the Gestalt movement,
from which all of their other ideas were developed. The central
message of Gestalt theory therefore is that the primary function of
perceptual processing is the generation of a miniature,
virtual-reality replica of the external world inside our head, and
that the world we see around us is not the real external world, but is
exactly that miniature internal replica (Lehar 2003). It is only in this context
that the elaborate model presented here begins to seem plausible. The mathematical form of the coplanarity interaction field can be
described as follows. Consider the field strength F due
to an element in the opaque state at some point in the volume of the
spatial matrix, with a certain surface orientation, depicted in
figure 17 A as a vector, representing the normal to the surface
encoded by that element. The strength of the field F
should peak within the plane at right angles to this normal vector
(depicted as a circle in figure 17 A) as defined in polar coordinates
by the function
Fa
= sin(a),
where
a
is the angle between the surface normal and some point in the field,
that ranges from zero, parallel to the normal vector, to p, in the opposite direction. The sine
function peaks at a = p/2, as shown
in Figure 17 B, producing an equatorial belt around the normal vector
as suggested schematically in cross-section in Figure 17 C, where the
gray shading represents the strength of the field. The strength of
the field should actually decay with distance from the element, for
example with an exponential decay function, as defined by the
equation
Far =
e-r2
sin(a)
as shown in Figure 17 D, where r is the radial distance from
the element. This produces a fading equatorial band, as suggested
schematically in cross-section in Figure 17 E. The equatorial belt
of the function described so far would be rather fat, resulting in a
lax or fuzzy coplanarity constraint, but the constraint can be
stiffened by raising the sine to some positive power P,
producing the equation
Far =
e-r2
sin(a)P
which will produce a sharper peak in the function as shown in Figure
17 F, producing a sharper in-plane field depicted schematically in
cross-section in Figure 17 G. In order to control runaway positive
feedback and suppress the uncontrolled proliferation of surfaces, the
field function should be normalized, in order to project inhibition
in directions outside the equatorial plane. This can be achieved with
the equation
Far =
e-r2
2 sin(a)P - 1
which has the effect of shifting the equatorial function
half way into the negative region as shown in Figure 17 H, producing
the field suggested in cross section in Figure 17 I.
The field described so far is un-oriented, i.e. it has a
magnitude, but no direction at any sample point (r,a). What is actually required is a field with a
direction, that would have maximal influence on adjacent elements
that are oriented parallel to it, i.e. elements that are coplanar
with it in both position and orientation. We can describe this
orientation of the field with the parameter q, that represents the orientation at which the
field F is sampled, expressed as an angle relative to the
normal vector; in other words, the strength of the influence F
exerted on an adjacent element located at a point (r,a) varies with the deviation q of that element from the direction parallel to
the normal vector, as shown in Figure 18, such that the maximal
influence is felt when the two elements are parallel, i.e. when q = 0, as in Figure 18 A, and falls off smoothly
as the other element's orientation deviates from that orientation as
in Figure 18 B and C. This can be expressed with a cosine function,
such that the influence F of an element on another element in
a direction a and separation r from
the first element, and with a relative orientation q would be defined by8.7 Bounding the Representation
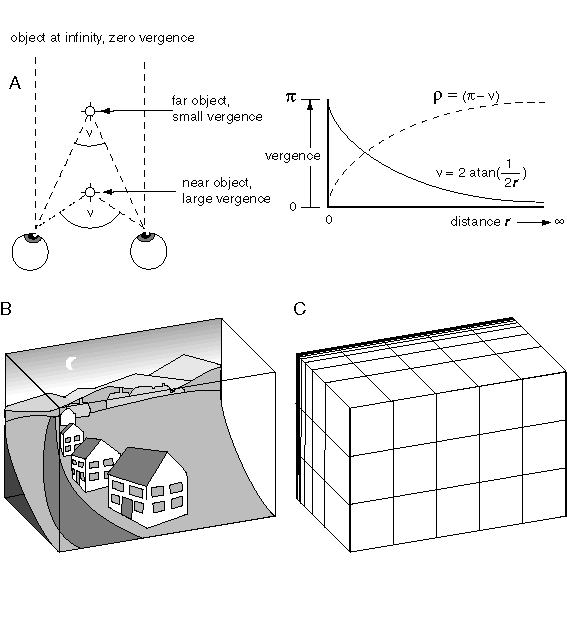
Figure 13
A: A vergence representation maps infinite distance into a finite
range. B: This produces a mapping reminiscent of a museum diarama.
C: The compressed reference grid in this compressed space defines
intervals that are perceived to be of uniform size.

Figure 14
A: An azimuth / elevation / vergence representation maps the infinity
of three-dimensional Euclidean space into a finite perceptual space.
B: The deformation of the infinite Cartesian grid caused by the
perspective transformation of the azimuth / elevation / vergence
representation. C: A view of a man walking down a road represented in
the perspective
distorted space. D: A section of the spherical space depicted in the
same format as the perspective space shown in figure 12.
8.8 Brain Anchoring
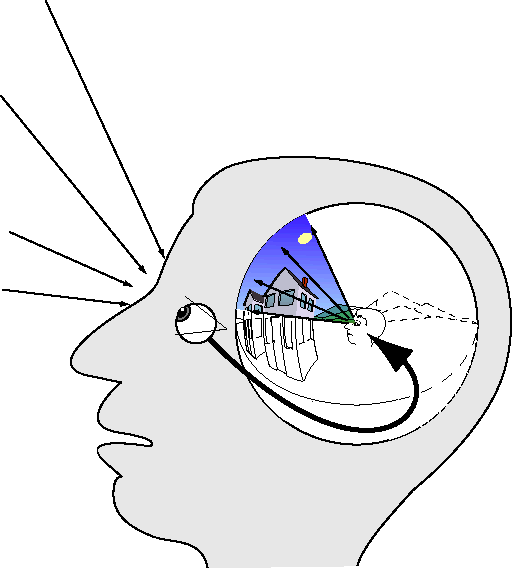
Figure 15
The image from the retina is projected into the perceptual sphere from
the center outward in the direction of gaze, as an inverse analog of
the cone of light that enters the eye in the external world, taking
into account eye, head, and body orientation in order to update the
appropriate portion of perceptual space.
9 Discussion
Figure 16
Perceptual interpolation in depth in illusory figure stereograms,
adapted from A: Carman et al. (1992), B: Kellman et al. (1991), and C:
Idesawa (1991). Opposite disparity percepts are achieved by binocular
fusion of either the first and second, or the second and third columns
of the figure.
10 Conclusion
Appendix
The Coplanarity Field

Figure 17
Progressive construction of the equation for the coplanarity field
from one element to another, as described in the text.
| Farq = e-r2 [2 sin(a)P - 1] | cos(q)Q | | (EQ 1) |
This cosine function allows the coplanar influence to propagate to near-coplanar orientations, thereby allowing surface completion to occur around smoothly curving surfaces. The tolerance to such curvature can also be varied parametrically by raising the cosine function to a positive power Q, as shown in Equation 1. So the in-plane stiffness of the coplanarity constraint is adjusted by parameter P, while the angular stiffness is adjusted by parameter Q. The absolute value on the cosine function in Equation 1 allows interaction between elements when q is between p/2 and p.
The orthogonality and occlusion fields have one less dimension of symmetry than the coplanarity field, and therefore they are defined with reference to two vectors through each element at right angles to each other, as shown in Figure 19 A. For the orthogonality field, these vectors represent the surface normals to the two orthogonal planes of the corner, while for the occlusion field one vector is a surface normal, and the other vector points within that plane in a direction orthogonal to the occlusion edge. The occlusion field G around the local element is defined in polar coordinates from these two vector directions, using the angles a and b respectively, as shown in Figure 19 A. The plane of the first surface is defined as for the coplanarity field, with the equation Gabr = e-r2 sin(a)P. For the occlusion field this planar function should be split in two, as shown in Figure 19 B to produce a positive and a negative half, so that this field will promote surface completion in one direction only, and will actually suppress surface completion in the negative half of the field. This can be achieved by multiplying the above equation by the sign (plus or minus, designated by the function sgn()) of a cosine on the orthogonal vector, i.e. Gabr = e-r2 sin(a)P sgn(cos(b)). Because of the negative half-field in this function, there is no need to normalize the equation. However the oriented component of the field can be added as before, resulting in the equation
| Gabrq = e-r2 [sin(a)P sgn(cos(b))] | cos(q)Q | | (EQ 2) |
Again, the maximal influence will be experienced when the two elements are parallel in orientation, i.e. when q = 0. As before, the orientation cosine function is raised to the positive power Q, to allow parametric adjustment of the stiffness of the coplanarity constraint.
The orthogonality field H can be developed in a similar manner, beginning with the planar function divided into positive and negative half-fields, i.e. with the equation Habr = e-r2 sin(a)P sgn(cos(b)) but then adding another similar plane from the orthogonal surface normal, producing the equation Habr = e-r2 [sin(a)P sgn(cos(b)) + sin(b)P sgn(cos(a))]. This produces two orthogonal planes, each with a negative half-field, as shown schematically in Figure 19 C. Finally, this equation must be modified to add the oriented component to the field, represented by the vector q, such that the maximal influence on an adjacent element will be experienced when that element is either within one positive half-plane and at one orientation, or is within the other positive half-plane and at the orthogonal orientation. The final equation for the orthogonality field therefore is defined by
| Habrq = e-r2 [sin(a)P sgn(cos(b)) | cos(q)Q | + [sin(b)P sgn(cos(a)) | cos(q)Q |] | (EQ 3) |
There is another aspect of the field-like interaction between elements that remains to be defined. Both the orthogonal and the occlusion states are promoted by appropriately aligned neighboring elements in the coplanar state. Orthogonal and occlusion elements should also feel the influence of neighboring elements in the orthogonal and occlusion states, because a single edge should have a tendency to become either an orthogonal corner percept, or an occlusion edge percept along its entire length. Therefore orthogonal or occlusion elements should promote like-states, and inhibit unlike-states in adjacent elements along the same corner or edge. The interaction between like-state elements along the edge will be called the edge-consistency constraint, and the corresponding field of influence will be designated E, while the complementary interaction between unlike-state elements along the edge is called the edge-inconsistency constraint, whose corresponding edge-inconsistency field will be designated I. These interactions are depicted schematically in Figure 20

The spatial direction along the edge can be defined by the product of the two sine functions sin(a) sin(b) defining the orthogonal planes, denoting the zone of intersection of those two orthogonal planes, as suggested in Figure 20 E. Again, this field can be sharpened by raising these sine functions to a positive power P, and localized by applying the exponential decay function. The edge consistency constraint E therefore has the form Eabr = e-r2 [sin(a)P sin(b)P]. As for the orientation of the edge-consistency field, this will depend now on two angles,q and f, representing the orientations of the two orthogonal vectors of the adjacent orthogonal or occlusion elements relative to the two normal vectors respectively. Both the edge-consistency and the edge-inconsistency fields, whether excitatory between like-state elements, or inhibitory between unlike-state elements, should peak when both pairs of reference vectors are parallel to the normal vectors of the central element, i.e. when q and f are both equal to zero. The full equation for the edge-consistency field E would therefore be
| Eabrqf = e-r2 [sin(a)P sin(b)P] cos(q)Q cos(f)Q | (EQ 4) |
where this equation is applied only to like-state edge or corner elements, while the edge-inconsistency field I would be given by
| Iabrqf = e-r2 [sin(a)P sin(b)P] cos(q)Q cos(f)Q | (EQ 5) |
applied only to unlike-state elements. The total influence R on an occlusion element therefore is calculated as the sum of the influence of neighboring coplanar, orthogonal, and occlusion state elements as defined by
| Rabrqf = Gabrqf + Eabrqf - Iabrqf | (EQ 6) |
and the total influence S on an orthogonal state element is defined by
| Sabrqf = Habrqf + Eabrqf - Iabrqf | (EQ 7) |
A two-dimensional visual edge has an influence on the three-dimensional interpretation of a scene, since an edge is suggestive of either a corner or an occlusion at some orientation in three dimensions whose two-dimensional projection coincides with that visual edge. This influence however is quite different from the local field-like influences described above, because the influence of a visual edge should penetrate the volumetric matrix with a planar field of influence to all depths, and should activate all local elements within the plane of influence that are consistent with that edge. Subsequent local interactions between those activated elements serves to select which subset of them should finally represent the three-dimensional percept corresponding to the two-dimensional image. For example, a vertical edge as shown in Figure 21 A would project a vertical plane of influence, as suggested by the light shading in Figure 21 A, into the depth dimension of the volumetric matrix, where it stimulates the orthogonal and occlusion states which are consistent with that visual edge. For example it would stimulate corner and occlusion states at all angles about a vertical axis, as shown in Figure 21 A, where the circular disks represent different orientations of the positive half-fields of either corner or occlusion fields. However a vertical edge would also be consistent with corners or occlusions about axes tilted relative to the image plane but within the plane of influence, for example about the axes depicted in Figure 21 B. The same kind of stimulation would occur at every point within the plane of influence of the edge, although only one point is depicted in the figure. When all elements consistent with this vertical edge have been stimulated, the local field-like interactions between adjacent stimulated elements will tend to select one edge or corner at some depth and at some tilt, thereby suppressing alternative edge percepts at that two-dimensional location at different depths and at different tilts. At equilibrium, some arbitrary edge or corner percept will emerge within the plane of influence as suggested in Figure 21 C, which depicts only one such possible percept, while edge consistency interactions will promote like-state elements along that edge, producing a single emergent percept consistent with the visual edge. In the absence of additional influences, for example in the isolated local case depicted in Figure 21 C, the actual edge that emerges will be unstable, i.e. it could appear anywhere within the plane of influence of the visual edge through a range of tilt angles, and could appear as either an occlusion or a corner edge. However when it does appear, it propagates its own field-like influence into the volumetric matrix, in this example the corner percept would propagate a planar percept of two orthogonal surfaces that will expand into the volume of the matrix, as suggested by the arrows in Figure 21 C. The final percept therefore will be influenced by the global pattern of activity, i.e. the final percept will construct a self-consistent perceptual whole, whose individual parts reinforce each other by mutual activation by way of the local interaction fields, although that percept would remain unstable in all unconstrained dimensions. For example the corner percept depicted in Figure 21 C would snake back and forth unstably within the plane of influence, rotate back and forth along its axis through a small angle, and flip alternately between the corner and occlusion states, unless the percept is stabilized by other features at more remote locations in the matrix.

Anstis S. & Howard I, (1978) A Craik-O'Brien-Cornsweet Illusion for Visual Depth. Vision Research 18 213-217.
Arnheim R. (1969) Art and Visual Perception: A Psychology of the Creative Eye. Berkeley, University of California Press.
Attneave F. (1971) Multistability in Perception. Scientific American 225 142-151.
Attneave F. (1982) Prägnanz and soap bubble systems: a theoretical exploration. in Organization and Representation in Perception, J. Beck (Ed.), Hillsdale NJ, Erlbaum.
Barlow H., Blakemore C., & Pettigrew J. (1967) The Neural Mechanism of Binocular Depth Discrimination. Journal of Physiology 193 327-342.
Barrow H. G. & Tenenbaum J. M. (1981) Interpreting Line Drawings as Three Dimensional Surfaces. Artificial Intelligence 17, 75-116.
Bisiach E., Capitani E., Luzatti C., & Perani D. (1981) Brain and Conscious Representation of Outside Reality. Neuropsychologia 19 543-552.
Bisiach E. & Luzatti C. (1978) Unilateral Neglect of Representational Space. Cortex 14 129-133.
Blank A. A. (1958) Analysis of Experiments in Binocular Space Perception. Journal of the Optical Society of America, 48 911-925.
Blumenfeld W. (1913) Untersuchungen über die Scheinbare Grösse im Sehraume. Zeitschrift für Psychologie 65 241-404.
Boring (1933) The Physical Dimensions of Consciousness. New York: Century.
Bressan P. (1993) Neon colour spreading with and without its figural prerequisites. Perception 22 353-361
Broad C. D. (1925) The mind and its place in nature. Routledge & Kegan Paul.
Brookes A. & Stevens K. (1989) The analogy between stereo depth and brightness. Perception 18 601-614.
Bruce V. & Green P. (1987) Visual Perception: Physiology, psychology, and ecology. Hillsdale NJ: Erlbaum.
Carman G. J., & Welch L. (1992) Three-Dimensional Illusory Contours and Surfaces. Nature 360 585-587.
Chalmers, D. J. (1995) Facing Up to the Problems of Consciousness. Journal of Consciousness Studies 2 (3) 200-219. Reprinted in "Toward a Science of Consciousness II, The Second Tucson Discussions and Debates". (1996) S. R. Hameroff, A. W. Kaszniak, & A. C. Scott (Eds.) MIT Press 5-28.
Charnwood J. R. B. (1951) Essay on Binocular Vision. London, Halton Press.
Churchland P. M. (1984) Matter and Consciousness: A contemporary introduction to the philosophy of mind. Cambridge MA: MIT Press.
Clark A. (1993) Sensory Qualities. Oxford UK: Oxford University Press.
Collett T. (1985) Extrapolating and Interpolating Surfaces in Depth. Proc. R. Soc. Lond. B 224 43-56.
Coren S., Ward L. M., & Enns J. J. (1994) Sensation and Perception. Ft Worth TX, Harcourt Brace.
Cornsweet T. N. (1970) Visual Perception. New York, Academic Press.
Crick F. & Koch C. (1990) Toward a Neurobiological Theory of Consciousness. Seminars in the Neurosciences 2: 263-275.
Crick F. (1994) The Astonishing Hypothesis: The Scientific Search for the Soul. New York: Scribners.
Davidson D. (1970) Mental Events. Oxford UK: Oxford University Press.
Dennett D. C. (1981) Two Approaches to Mental Images. In N. Block (Ed.) Imagery. Cambridge MA: MIT Press, 87-107
Dennett D. C. (1991) Consciousness Explained. Boston, Little Brown & Co.
Dennett D. C. (1992) `Filling In' Versus Finding Out: a ubiquitous confusion in cognitive science. In Cognition: Conceptual and Methodological Issues, Eds. H. L. Pick, Jr., P. van den Broek, & D. C. Knill. Washington DC.: American Psychological Association.
Denny-Brown D. & Chambers R. A. (1958) The Parietial Lobe and Behavior. A. Res Nerv Ment Dis 36, 35-117.
de Renzi E. (1982) Disorders of Space Exploration and Cognition. New York: John Wiley.
Drake, D. (1920) The approach to critical realism. In Essays in critical realism: A co-operative study of the problem of knowledge, eds. Drake, D., Lovejoy, A. O., Pratt, J. B., Rogers, A. K., Santayana, G., Sellars, R. W., & Strong C. A. Gordian Press: 3-32
Drake, D., Lovejoy, A. O., Pratt, J. B., Rogers, A. K., Santayana, G., Sellars, R. W., & Strong C. A. Essays in critical realism: A co-operative study of the problem of knowledge. Gordian Press.
Earle D. C. (1998) On the Roles of Consciousness and Representations in Visual Science. Behavioral & Brain Sciences 21 (6), pp 757-758, commentary on Pessoa et al. (1998).
Eckhorn R., Bauer R., Jordan W., Brosch M., Kruse W., Munk M., Reitboeck J. (1988) Coherent Oscillations: A Mechanism of Feature Linking in the Visual Cortex? Biol. Cybern. 60 121-130.
Feigl, H. (1958) The "mental" and the "physical". University of Minnesota Press.
Foley J. M. (1978) Primary Distance Perception.In: Handbook of Sensory Physiology, Vol VII Perception. R. Held, H. W. Leibowitz, & HJ. L. Tauber (Eds.) Berlin: Springer Verlag, pp 181-213.
Gibson J. J. (1972) A Theory of Direct Visual Perception. In: The Psychology of Knowing. (J. R. Royce & W. W. Rozeboom (Eds.), Gordon & Breach.
Gibson J. J. (1979) The Ecological Approach to Visual Perception. Houghton Mifflin.
Gibson J. J. & Crooks L. E. (1938) A Theoretical Field-Analysis of Automobile Driving. The American Journal of Psycholgy 51 (3) 453-471.
Gillam B. (1971) A Depth Processing Theory of the Poggendorf Illusion. Perception & Psychophysics 10, 211-216.
Gillam, B. (1980) Geometrical Illusions. Scientific American 242 102-111.
Graham C. H. (1965) Visual Space Perception. in C. H. Graham (Ed.) Vision and Visual Perception, New York, John Wiley 504-547.
Green M. & Odum V. J. (1986) Correspondence Matching in Apparent Motion: Evidence for Three Dimensional Spatial Representation. Science 233 1427-1429.
Gregory R. L. (1963) Distortion of Visual Space as Inappropriate Constancy Scaling. Nature 199, 678-679.
Grossberg, S. (1987) Cortical dynamics of three-dimensional form, color, and brightness perception: II. Binocular theory. Perception & Psychophysics 41: 117-58.
Grossberg S. (1990) Neural FAÇADE: Visual representations of static and moving Form-And-Color-And-Depth, Mind and Language 5 (Special Issue on Understanding Vision) 411-456.
Grossberg, S. (1994) 3-D vision and figure-ground separation by visual cortex. Perception & Psychophysics 55: 48-120.
Grossberg S, & Mingolla E, (1985) "Neural Dynamics of Form Perception: Boundary Completion, Illusory Figures, and Neon Color Spreading" Psychological Review 92 173-211.
Grossberg S, & Todorovic D, (1988) "Neural Dynamics of 1-D and 2-D Brightness Perception: A Unified Model of Classical and Recent Phenomena" Perception and Psychophysics 43, 241-277.
Harrison S. (1989) A New Visualization on the Mind-Brain Problem: Naive Realism Transcended. In J. Smythies & J. Beloff (Eds.) The Case for Dualism. Charlottesville: University of Virginia.
Heckenmuller E. G. (1965) Stabilization of the Retinal Image: A Review of Method, Effects, and Theory. Psychological Bulletin 63 157-169.
Heelan P. A. (1983) Space Perception and the Philosophy of Science. Berkeley. University of California Press.
Heilman K. M. & Watson R. T. (1977) The Neglect Syndrome - A unilateral defect of the orienting response. In S. Harnad, R. W. Doty, L. Goldstein, J. Jaynes, & G. Krauthamer (Eds.) Lateralization in the Nervous System. New York: Academic Press.
Heilman K. M., Watson R. T. & Valenstein E. (1985) Neglect and Related Disorders. In K. M. Heilman & E. Valenstein (Eds.) Clinical Neuropsychology. New York: Oxford University Press.
Helmholtz H. (1925) Physiological Optics. Optical Society of America 3 318.
Hillebrand F. (1902) Theorie der Scheinbaren Grösse bei Binocularem Sehen. Denkschr. Acad. Wiss. Wien (Math. Nat. Kl.), 72 255-307.
Hochberg J. & Brooks V. (1960) The Psychophysics of Form: Reversible Perspective Drawings of Spatial Objects. American Journal of Psychology 73 337-354.
Hoffman D. D. (1998) Visual Intelligence: How We Create What We See. New York: W. W. Norton.
Idesawa M. (1991) Perception of Illusory Solid Object with Binocular Viewing. Proceedings IJCNN
Indow T. (1991) A Critical Review of Luneberg's Model with Regard to Global Structure of Visual Space. Psychological Review 98, 430-453.
Julesz B. (1971) Foundations of Cyclopean Perception. Chicago, University of Chicago Press.
Kanizsa G, (1979) Organization in Vision. New York, Praeger.
Kant I. (1781 / 1991) Critique of Pure Reason. Vasilis Politis (Ed.) London: Dent.
Kaufman (1974) Sight and Mind. New York, Oxford University Press.
Kellman P. J., & Shipley T. F. (1991) A Theory of Visual Interpolation in Object Perception. Cognitive Psychology 23 141-221.
Kellman P. J., Machado L. J., Shipley T. F., & Li C. C. (1996) Three-Dimensional Determinants of Object Completion. Annual Review of Vision and Ophthalmology (ARVO) abstracts, 3133 37 (3) p. S685.
Kinsbourne M. (1987) Mechanisms of Unilateral Neglect. In M. Jeannerod (Ed.) Neurophysiological and Neuropsychological Aspects of Spatial Neglect. Amsterdam: North-Holland.
Kinsbourne, M. (1993). Orientational bias model of unilateral neglect:Evidence from attentional gradients within hemispace. In I.H. Robertson & J.C. Marshall (Eds), Unilateral neglect: clinical and experimental studies (pp. 63-86). Hove, UK: Erlbaum.
Koffka K, (1935) Principles of Gestalt Psychology. New York, Harcourt Brace & Co.
Köhler W. & Held R. (1947) The Cortical Correlate of Pattern Vision. Science 110: 414-419.
Köhler W. (1969) The Task of Gestalt Psychology. Princeton NY. Princeton University Press.
Köhler W. (1971) A Task For Philosophers. In: The Selected Papers of Wolfgang Koehler, Mary Henle (Ed.) Liveright, New York. pp 83-107.
Kolb B. & Whishaw I. Q. (1996) Fundamentals of Human Neuropsychology. W. H. Freeman, p. 247-276.
Kosslyn S. M. (1975) Information Representation in Visual Images. Cognitive Psychology 7 341-370.
Kosslyn S. M. (1980) Image and Mind. Cambridge MA, Harvard University Press.
Kosslyn S. M. (1994) Image and Brain: The Resolution of the Imagery Debate. Cambridge MA, MIT Press.
Kuhn T. S. (1970) The Structure of Scientific Revolutions. Chicago: Chicago University Press.
Lehar S. (2002) Directional Harmonic Theory: A Computational Gestalt
Model to Account for Illusory Contour and Vertex Formation. Submitted
Perception, August 2001. Also available at:
.//webstuff/dirhr1/dirhr1.html
Lehar S. (2003) The World In Your Head: A Gestalt view of the mechanism
of conscious experience. Mahwah NJ, Erlbaum. Information available at:
.//webstuff/book/WIYH.html
Ládavas E., Berti A., Ruozzi E., & Barboni F. (1997) Neglect as a Deficit Determined by an Imbalance Between Spatial Representations. Experimental Brain Research 116, 493-500.
Lesher G. W. (1995) Illusory Contours: Toward a Neurally Based Perceptual Theory. Psychonomic Bulletin and Review 2:279-321.
Llinas R. R., Ribary U., Joliot M., & Wang X. -J (1994) Content and Context in Temporal Thalamocortical Binding. In G. Buzsaki, R. R. Llinas, & W. Singer (Eds.) Temporal Coding in the Brain. Berlin: Springer-Verlag.
Luneburg R. K. (1950) The Metric of Binocular Visual Space. Journal of the Optical Society of America, 40 627-642.
Marshall J. C. & Halligan P. W. (1995) Seeing the Forest but only Half the Trees? Nature 373, 521-523.
Marr D. & Poggio T. (1976) Cooperative Computation of Stereo Disparity. Science 194 283-287.
Mitchison G, (1993) The neural representation of stereoscopic depth contrast. Perception 22 1415-1426
Movshon J. A., Adelson E. H., Gizzi M. S., & Newsome W. T. (1986) The Analysis of Moving Patterns. In C. Chagas, R. Gattass, & C. Cross (Eds.) Pattern Recognition Mechanisms, 112-151. Berlin: Springer Verlag.
Müller G. E. (1896) Zur Psychophysik der Gesichtsempfindungen. Zeitschrift für Psychologie 10.
Nagel T. (1974) What Is It Like to Be a Bat? Philosophical Review 83 435-450
Opie, J. (1999) Gestalt theories of cognitive representation and processing. Psycoloquy 10(021)
O'Regan K. J., (1992) Solving the `Real' Mysteries of Visual Perception: The World as an Outside Memory. Canadian Journal of Psychology 46 461-488.
Palmer, S.E. (1992) Modern theories of Gestalt perception. In: G.W.Humphreys (ed.) Understanding Vision. Blackwell.
Palmer, Steven E. (1999) Color, Consciousness, and the Isomorphism Constraint Behavioral and Brain Sciences 22 (6): 1-21.
Pessoa L., Thompson E., & Noë A. (1998) Finding Out About Filling-In: A guide to perceptual completion for visual science and the philosophy of perception. Behavioral and Brain Sciences 21, 723-802.
Pinker S. (1980) Mental Imagery and the Third Dimension. Journal of Experimental Psychology 109 354-371.
Pinker S. (1988) A Computational Theory of the Mental Imagery Medium. In: M. Denis, J. Engelkamp, J. T. E. Richardson (Eds.) Cognitive and Neuropsychological Approaches to Mental Imagery. Boston, Martinus Nijhoff.
Price H. H. (1932) Perception. London: Methuen & Co. Ltd.
Ramachandran V. S. & Anstis S. M. (1986) The Perception of Apparent Motion. Scientific American 254 80-87.
Ramachandran V. S. (1992) Filling in Gaps in Perception: Part 1 Current Directions in Psychological Science 1 (6) 199-205
Read, S.J., Vanman, E.J. & Miller, L.C. (1997) Connectionism, Parallel Constraint Satisfaction Processes, and Gestalt Principles: (Re)Introducing Cognitive Dynamics to Social Psychology. Personality and Social Psychology Review, 1(1):26- 53.
Revonsuo A. (1995) Consciousness, Dreams, and Virtual Realities. Philosophical Psychology 8 (1) 35-58.
Revonsuo A. (1998) Visual Perception and Subjective Visual Awareness. Open peer commentary to Pessoa et al. (1998) pp 769-770.
Rock I, & Brosgole L. (1964) Grouping Based on Phenomenal Proximity. Journal of Experimental Psychology 67 531-538.
Rosenberg G. (2002) A Place for Consciousness: The theory of natural
individuals. (submitted for publication) Also available at:
http://ai.uga.edu/~ghrosenb/book.html
Russell B. (1927) Philosophy. New York: W. W. Norton.
Sacks, O. (1985) The Man Who Mistook His Wife For a Hat. New York, Harper & Row. p. 77-79
Searle, J. R. (1980) "Minds, Brains, and Programs." Behavioral and Brain Sciences 3, 417-424.
Searle J. R. (1992) The Rediscovery of Mind. Cambridge MA: The MIT Press.
Searle J. R. (1997) The Mystery of Consciousness. New York: New York Review.
Shannon C. E. (1948) A Mathematical Theory of Communication. Bell Systems Technical Journal 27: 379-423.
Shepard R. N. (1981) Psychophysical Complementarity. In M. Kubovy & J. Pomeranz (Eds.) Perceptual Organization. Mahwah NJ: Erlbaum.
Shepard R. N. & Chipman S. (1970) Second-Order Isomorphism of Internal Representations: Shapes of States. Cognitive Psychology 1, 1-17.
Shepard R. N. & Metzler J. (1971) Mental Rotation of Three-Dimensional Objects. Science 171 701-703.
Singer W. (1999) Neuyronal Synchrony: A versatile code for the definition of relations? Neuron 24: 49-65.
Singer W. & Gray C. (1995) Visual Feature Integration and the Temporal Correlation Hypothesis. Annual Review of Neuroscience 18, 555-586.
Singh M. & Hoffman D. D. (1998) Active Vision and the Basketball Problem. Behavioral & Brain Sciences 21 (6), pp 772-773, commentary on Pessoa et al. (1998).
Smythies J. R. (1989) The Mind-Brain Problem. In: J. R. Smythies & J. Beloff (Eds) The Case For Dualism. Charlottesville: University of Virginia Press.
Smythies J. R. (1994) The Walls of Plato's Cave: the science and philosophy of brain, consciousness, , and perception. Aldershot UK: Avebury.
Takeichi H, Watanabe T, Shimojo S, (1992) Illusory occluding contours and surface formation by depth propagation. Perception 21 177-184
Tausch, R. (1954) Optische Täuschungen als artifizielle Effekte der Gestaltungs-prozesse von Grössen und Formenkonstanz in der natürlichen Raumwahrnehmung. Psychologische Forschung, 24, 299-348.
Tse P. U. (1999a) Illusory Volumes from Conformation. Perception (in press).
Tse P. U. (1999b) Volume Completion. Cognitive Psychology (submitted)
Vallar G. (1998) Spatial Hemineglect in Humans. Trends in Cognitive Sciences, 2 (3) 87-96.
Velmans M. (1990) Consciousness, Brain and the Physical World. Philosophical Psychology 3 (1) 77-99.
Ware C. & Kennedy J. M. (1978) Perception of Subjective Lines, Surfaces and Volumes in 3-Dimensional Constructions. Leonardo 11 111-114.
Westheimer G. & Levi D. M. (1987) Depth Attraction and Repulsion of Disparate Foveal Stimuli. Vision Research 27 (8) 1361-1368.
Yarbus A. L. (1967) Eye Movements and Vision. New York: Plenum Press.
Zucker S. W., David C., Dobbins A., & Iverson L. 1988 "The Organization of Curve Detection: Coarse Tangent Fields and Fine Spline Coverings". Proceedings: Second International Conference on Computer Vision, IEEE Computer Society, Tampa FL 568-577.